Organizing committee of Beijing-Saint Petersburg Mathematics Colloquium
(1) Huijun Fan (WHU & SRMC, symplectic geometry and mathematical physics, geometric analysis)
(2) Feimin Huang (CAS, institute of applied mathematics, partial differential Equation, fluid mechanics)
(3) Sergey Kryzhevich (SPBU, differential and difference equations, dynamical systems, chaotic dynamics, structural stability, hyperbolic theory)
(4) Nikolay Mnev (PDMI RAS, SPBU, Algebraic Topology, Geometric Topology and Combinatorial Geometry)
(5) Victor Petrov (SPBU, Algebraic geometry)
(6) Wenyuan Yang (BICMR, geometric group theory and Kleinian groups)
(7) Xinyi Yuan (BICMR, number theory and arithmetic geometry)
*******
The lecture announcements will be continually updated. The arrangement of the upcoming lectures is as follows:
*******
Lecture Series 79 —— June 19, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA460CD2AF8CA94BB787A3B3EBC8D0AD83
Lecture 1 —— Semiclassical computation of non-adiabatic quantum dynamics
Speaker: Shi Jin (Shanghai Jiao Tong University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We develop efficient computational methods for oscillatory hyperbolic systems arising in non-adiabatic quantum dynamics involving band-crossing. Band-crossing is a quantum dynamical behavior that contributes to important physics and chemistry phenomena such as quantum tunneling, Berry connection, chemical reaction etc. In this talk, we will discuss our works in developing semiclassical methods for band-crossing in surface hopping. For such systems, which are hyperbolic but with oscillatory terms modeling non-adiabatic quantum transitions between different energy bands, we will introduce a nonlinear geometric optics method based "asymptotic-preserving" method that is accurate uniformly for all wave numbers, including the problem with random uncertain band gaps.
Bio: Shi Jin is the Director of Institute of Natural Sciences, and Chair Professor of Mathematics, at Shanghai Jiao Tong University. He also serves as a co-director of the Shanghai National Center for Applied Mathematics, director of Ministry of Education Key Lab on Scientific and Engineering Computing, and director of Shanghai Jiao Tong University Chongqing Artificial Intelligence Institute.
He is an inaugural Fellow of the American Mathematical Society (AMS) (2012), a Fellow of Society of Industrial and Applied Mathematics (SIAM) (2013), an inaugural Fellow of the Chinese Society of Industrial and Applied Mathematics (CSIAM) (2020), and an Invited Speaker at the International Congress of Mathematicians in 2018. In 2021 he was elected a Foreign Member of Academia Europaea and a Fellow of European Academy of Sciences. In 2024 he was awarded a Shanghai Natural Science Prize (first class).
His research interests include kinetic theory, hyperbolic conservation laws, quantum dynamics, uncertainty quantification, interacting particle systems, computational fluid dynamics, machine learning and quantum computing, etc.
Lecture 2 —— The number of spanning trees in a graph
Speaker: Danila Cherkashin (Institute of Mathematics and Informatics of Bulgarian Academy of Sciences)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The subject of the talk lies at the intersection of combinatorics, linear algebra and complex analysis.
Calculating the number of spanning trees in a graph goes back to the celebrated result of Kirchhoff (1847), who connected the number of trees and the Laplacian matrix. However, as was shown by Cayley (1889), there is a bijection between the summands of (x_1 + x_2 + ... + x_n)^(n-2) and the trees in a complete n-vertex graph (and in particular the number of trees is n^(n-2)). Here a tree T corresponds to a monomial in which degree of x_i is equal to the degree of vertex i in T minus 1. In other words the vertex spanning enumerator polynomial of a complete graph has a linear factorization. We discuss all strengthening of these results that I know, in particular the following result by C., Petrov and Prozorov (2023).
The following statements are equivalent.
2) The vertex spanning enumerator polynomial of G factors into linear terms.
3) The vertex spanning enumerator polynomial of G is real stable (i.e., it does not vanish when substituting any variables from the open upper complex half-plane).
We finish with open questions.
Bio: Dr. Cherkashin is a brilliant expert in Graph Theory, Combinatorics and Dynamical Systems. He graduated from Saint Petersburg State University in 2015, got his PhD in 2018 (Saint Petersburg Department of Steklov Mathematical Institute) and habilitated at IMI BAS in 2024. Being graduated, he has worked at Department of Mathematics and Computer Sciences from Saint Petersburg State University before moving to IMI BAS in 2022.
*********************
Lecture Series 78 —— June 5, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAE9C3EDD0C28541F39DF516874C9755A7
Lecture 1 —— Gromov-Witten invariants of elliptic curves revisited
Speaker: Jie Zhou (Yau Mathematical Sciences Center, Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Elliptic curves play an important role in understanding the modern aspects of enumerative geometry. In this talk I will start by reviewing some results of Okounkov-Pandharipande and Bloch-Okounkov on the generating series of Gromov-Witten invariants of elliptic curves. I will then report my recent results on this topic from the perspective of the mirror elliptic curve. These include:
1. a formulation of the (all-genus) generating series as period integrals on the configuration space of the mirror curve,
2. new combinatorial formulas for the generating series,
3. a connection between a slightly modified but equivalent version of the generating series and mixed Hodge structures on the cohomology of configuration spaces of the mirror curve.
The talk is based on my recent works arXiv:2304.03912, arXiv:2310.08018, arXiv:2305.12362.
Bio: Jie Zhou is an associate professor at the Yau Mathematical Sciences Center of Tsinghua University. He obtained his PhD degree from Harvard University in 2014 and did postdoctoral researchers at Perimeter Institute in Canada and University of Cologne in Germany, before joining Tsinghua University in 2018. His research interest lies at the intersection of enumerative geometry, mirror symmetry, and modular forms.
Lecture 2 —— A Model of Optimal Exploitation of Renewable Resources and Related Issues in Optimal Control Theory
Speaker: Sergei Aseev (Steklov Mathematical Institute of Russian Academy of Sciences)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: This presentation focuses on a basic model for the optimal exploitation of renewable resources, alongside pertinent topics in the mathematical theory of optimal control. The model is framed as an infinite horizon optimal control problem. We introduce a recently developed complete version of the Pontryagin maximum principle applicable to such problems. Additionally, we provide an analytical exploration of the problem under consideration. Finally, we discuss the economic interpretation of the results obtained.
Bio: Sergei Mironovich Aseev is a Principal Researcher at Steklov Mathematical Institute, Doctor of Sciences in Mathematics, and Corresponding Member of Russian Academy of Sciences.
His research interests include optimal control, dynamic systems, differential inclusions, multivalued mappings, mathematical economics. S.M. Aseev obtained theorems on the approximation of semi-continuous multivalued mappings by continuous ones, and proposed an axiomatic approach to the study of subset spaces and functional spaces of multivalued mappings. Methods were developed for studying non-smooth optimal control problems for differential inclusions using their approximations by classical smooth optimal control problems, and an optimal control problem for a differential inclusion with a phase constraint was investigated.
Prof. Aseev graduated from Moscow State University in 1980, has got his PhD in 1983 and habilitated in 1998. In 2001–2004 he worked as a research fellow at the International Institute for Applied Systems Analysis, Laxenburg, Austria.
He combines his work at Steklov institute with teaching at Moscow State University. Prof. Aseev is an author of multiple highly quoted books and research papers, and laureate of several prestigious prizes.
*********************
Lecture Series 77 —— May 22, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA570E9CC0FC0D4996A2503A8D4E44B863
Lecture 1 —— Stability theory of singularities
Speaker: Chenyang Xu (Princeton University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Over the past decade, a local theory of K-stability for Kawamata log terminal (klt) singularities has emerged. This theory studies minimizers of a normalized volume function and associates a K-semistable Fano cone to any given klt singularity. In many cases, this construction significantly generalizes the notion of the metric tangent cone for singular Kähler–Einstein spaces. The lecture is intended for a broad mathematical audience.
Bio: Chenyang Xu, born in 1981 in Chongqing, China, is a distinguished mathematician specializing in algebraic geometry. After earning his Ph.D. from Princeton University in 2008 under the mentorship of János Kollár, Xu held academic positions at institutions including MIT, the University of Utah, and Peking University, before returning to Princeton as a professor. His research focuses on birational geometry, the minimal model program, and the K-stability of Fano varieties. Xu's contributions have been recognized with several prestigious awards: the 2016 ICTP Ramanujan Prize for his work in birational geometry, the 2017 Future Science Prize in Mathematics and Computer Science for fundamental contributions to the field, the 2019 New Horizons in Mathematics Prize for advances in the minimal model program and moduli of algebraic varieties, and the 2021 Frank Nelson Cole Prize in Algebra for developing an algebraic theory of moduli for K-stable Fano varieties and pioneering new approaches to singularities in the minimal model program using K-stability. He was also an invited speaker at the 2018 International Congress of Mathematicians (ICM).
Lecture 2 —— Complexity of Infinite Words
Speaker: Svetlana Puzynina (St. Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Complexity of infinite words is a widely studied field in combinatorics on words. A classical notion of a complexity of an infinite word is defined as a function counting, for each n, the number of its distinct factors (or blocks of consecutive letters) of length n. In their 1938 seminal paper on symbolic dynamics, Morse and Hedlund gave a relation between factor complexity and periodicity in infinite words; namely, they proved that each aperiodic infinite word w has factor complexity at least n+1 for each length n. They further showed that an infinite word w has factor complexity n+1 for each length n if and only if w is binary, aperiodic and balanced, i.e., w is a Sturmian word. In the talk, we will consider various modifications of the notion of a complexity of infinite words and generalizations of Morse and Hedlund theorem.
Bio: Svetlana Puzynina is an associate professor at Saint Petersburg State University, Russia. She obtained her PhD from the Sobolev Institute of Mathematics in 2008 and spent several years at University of Turku, Finland, Ecole Normale Supérieure de Lyon, Université Paris Diderot, France. Svetlana's main field of expertise is combinatorics on words and its interactions with other fields of mathematics including symbolic dynamics, Ramsey theory and automata.
*********************
Lecture Series 76 —— May 8, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA38CD1D8C254B4141A5D2C199525F23B1
Lecture 1 —— $\Phi^4_3$ Theory from many-body quantum Gibbs states
Speaker: Rongchan Zhu (Beijing Institute of Technology)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We derive the $\Phi^4_3$ measure on the torus as a rigorous limit of the quantum Gibbs state of an interacting Bose gas, where the limiting classical measure describes the critical behavior of the Bose gas just above the Bose--Einstein phase transition. Since the quantum problem is typically formulated using a nonlocal interaction potential, a key challenge is to approximate the local $\Phi^4_3$ theory by a Hartree measure with a nonlocal interaction. This requires uniform estimates on the Hartree measure, which are achieved using techniques from recent development on stochastic quantization and paracontrolled calculus. The connection to the quantum problem is then established by applying the variational approach and deriving a quantitative convergence of the quantum correlation functions to those of the Hartree classical field.
Bio: Rongchan Zhu is a Professor at Beijing Institute of Technology. She earned her Ph.D. in 2012 from both the Academy of Mathematics and Systems Science, Chinese Academy of Sciences, and Bielefeld University in Germany. Her research focuses on Stochastic Partial Differential Equations (SPDEs), with particular emphasis on singular SPDEs, stochastic fluid equations, and the study of quantum field models via stochastic quantization methods.
Lecture 2 —— Quantization of the theory of topological insulators
Speaker: Armen Sergeev (Steklov Mathematical Institute of Russian Academy of Sciences)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Topological insulators are the solid bodies having a broad energy gap stable under small deformations. This motivates the usage of topological methods for their study.
A key role in the theory of solid states is played by their symmetry groups. Kitaev has pointed out that there is a relation between the symmetries of solid bodies and Clifford algebras. According to this observation the quantization of topological insulators should reduce to the theory of irreducible representations of Clifford algebras.
The next important step was done by Kennedy and Zirnbauer who introduced the notion of pseudosymmetries. While the algebra of observables of a topological insulator is generated by Hamiltonians satisfying commutation relations with symmetry operators, the quantum obsrvables are given by the complex sstructures on the Nambu space satisfying anticommutation relations with pseudosymmetries. This correspondence determines the quantization of topological insulators.
Bio: Armen Glebovich Sergeev is an outstanding Russian mathematician whose interests include complex analysis and complex geometry of tubular domains, pseudoholomorphic curves, matrix domains of holomorphy, geometric quantization, Seiberg-Witten equations, vortex equations, infinite-dimensional Kähler manifolds and harmonic mappings of compact Riemann surfaces into Kähler manifolds. Besides, studies the universal Teichmüller space, which contains the classical Teichmüller spaces of compact Riemann surfaces of finite genus as complex submanifolds.
Prof. Sergeev graduated from the Faculty of Mechanics and Mathematics of Moscow State University in 1971. Four years later, he defended his PhD thesis on the topic “Multidimensional factorization problem and multiplicative theory of hyperfunctions” being supervised by Prof. A. G. Kostyuchenko.
Since 1982, he has been working at the Steklov Mathematical Institute (MIAN), now being Deputy Director of the institute.
In 1989, Armen Sergeev became Doctor of Physical and Mathematical Sciences. He teaches at the Faculty of Mechanics and Mathematics, as a professor of the Department of Function Theory and Functional Analysis (1994).
Besides, Prof. Sergeev is a Member of the Board of the Moscow Mathematical Society (MMS), Editor of the MMS Proceedings, Deputy Editor-in-Chief of the journal “Izvestiya RAS. Mathematical Series”. Honorary Doctor of Heńań University (Heńań, China) and foreign Member of the National Academy of Sciences of the Republic of Armenia (2014).
*********************
Lecture Series 75 —— April 24, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAB3B3DC4E6CE34975BE5B67926113E84F
Lecture 1 —— Some recent progress on the 3D kinetic shear flow for the Boltzmann equation in the hydrodynamic limit
Speaker: Shuangqian Liu (Central China Normal University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, I will present our recent studies on the hydrodynamic limits of the Boltzmann equation, focusing on the rigorous derivation of Couette flow from the Boltzmann equation to the incompressible Navier-Stokes system. Central to our approach are novel anisotropic estimates within the Wiener algebra function space, which enable a precise treatment of the kinetic-to-fluid transition. Additionally, I will discuss extensions to more general shear flows, including the Kolmogorov flow.
Bio: Shuangqian Liu is currently a professor of School of Mathematics and Statistics at the Central China Normal University. He received his PhD from Wuhan University in 2009, he was a postdoctor in the department of Mathematics at CUHK from 2013 to 2014. His research interest is analysis of PDEs with focus on the Boltzmann equation in kinetic theory as well as related fluid dynamic equations. He was supported by outstanding youth grand from NSFC in 2023.
Lecture 2 —— Galerkin method and implementation of functional type a posteriori error estimates with black-box solvers for Linear Elasticity
Speaker: Maksim Frolov (Peter the Great St. Petersburg Polytechnic University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A posteriori error estimates provide an explicit accuracy control for numerical solutions of boundary-value problems for Partial Differential Equations.
It is an important and practically significant area of research in applied mathematics. Regardless of the fact that the classical methods of a posteriori error control are investigated very intensively for several decades, functional-type a posteriori estimates are very promising. This approach is fully reliable and it can be used to solvers with some hidden details of numerical implementations. Such estimates are known for many problems of the elasticity theory. However, as follows from the work of Prof. S. Repin and Dr. A. Muzalevsky, when implementing a posteriori estimates of the functional type, the use of classical approximations of the Finite Element Method leads to a growing overestimation of the absolute value of the error. Later, in the work of M. Frolov, this effect is highlighted more transparently. It is shown that the use of approximations that are more natural for mixed finite element methods avoids a growing overestimation of the absolute error value with mesh refinements. Comprehensive numerical testing and justification of this approach are provided in joint papers with Dr. M. Churilova and Ph.D. student D. Petukhov. In particular, a comparative analysis is performed for zero-order and first-order Raviart-Thomas finite elements implemented in MATLAB by D. Petukhov. For plane problems of linear elasticity, it is shown that the use of the first-order Raviart-Thomas approximation significantly reduces an overestimation of the absolute error value.
Bio: Maksim Evgenyevich Frolov has received his Ph.D. in Applied Mathematics in 2004 (thesis “A posteriori error estimates for approximate solutions to variational problems for elliptic equations of divergent type”) and D.Sci. in 2015 (with work “Functional methods and its implementation to a posteriori error control in Linear Elasticity”). From 2016 to 2024 he was the Director of the Institute of Physics and Mechanics at Peter the Great St. Petersburg Polytechnic University (the historical name PhysMech was returned in 2021; for the period 2013-2021 — the Institute of Applied Mathematics and Mechanics). At present he is a professor of this institute.
His research interests include reliable modelling, a posteriori error estimates, numerical methods for PDE’s (Finite Element Methods), computational mechanics. Prof. Frolov constructed new reliable error estimates for several practically important models in Linear Elasticity theory: the biharmonic equation, Timoshenko beams, Reissner-Mindlin plates (joint work with Prof. S. Repin from PDMI RAS, Russia and Prof. P. Neittaanmäki from University of Jyväskylä, Finland), the Cosserat theory of elasticity in 2D (joint work with Prof. S. Repin) and 3D. He also introduced new methods of implementation of the functional approach to a posteriori error control in adaptive algorithms in 2D based on Raviart-Thomas and Arnold-Boffi-Falk approximations.
*********************
Lecture Series 74 —— April 10, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA64CC8BDC1E5D4AB59C73516C8382200E
Lecture 1 —— Anosov flows on Dehn surgeries on the figure-eight knot
Speaker: Bin Yu (Tongji University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, we explore the classification of three-dimensional Anosov flows, with particular focus on hyperbolic 3-manifolds arising from Dehn surgeries on the figure-eight knot. We will also discuss foundational concepts in Anosov flows, survey recent advances in understanding orbital equivalence in this geometric setting, and highlight key open problems in the field.
Bio: Dr. Bin Yu obtained his Ph.D. in Mathematics from Peking University in 2007 and currently serves as a professor at Tongji University. His research bridges foundational investigations in manifold structures with the classification theory of dynamical systems, specializing in geometric topology and low-dimensional dynamics.
Lecture 2 —— Rectangular diagrams of links, surfaces, and foliations
Speaker: Ivan Dynnikov (Steklov Mathematical Institute of RAS, MSU)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The formalism of rectangular diagrams provides a convenient framework for representing links and surfaces in the three-sphere in a consistent manner. They appear to have a strong connection with contact topology, as well as nice combinatorial properties, which make them useful for solving algorithmic and classification problems of knot theory. In particular, they allow to construct a simple algorithm for recognizing the unknot and to solve the problem of algorithmic classification of Legendrian and transverse links. One can also use rectangular diagrams to obtain explicit presentations of finite depth taut foliations in link complements.
Bio: Ivan Alekeevich Dynnikov is a leading researcher at Steklov Mathematical Institute and a professor of Moscow State University and a worldwide known expert in geometry and topology. His research interests include low-dimensional topology, dynamical systems, graph theory, mathematical physics, foliations, braid theory and knot theory. He has received his DS/Habilitation degree in 2007 and a honorable degree of Professor of Russian Academy of Sciences in 2016.
Prof. Dynnikov solved several famous problems, for instance:
- he constructed a monotone simplification algorithm for recognizing a trivial knot;
- proved Jones's conjecture (jointly with M. V. Prasolov);
- solved S. P. Novikov's problem on plane sections of 3-periodic surfaces;
- constructed a new discrete analogue of a complex structure on a plane (jointly with S. P. Novikov).
*********************
Lecture Series 73 —— March 27, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAAB87AD03C89945D0A5F4702518F7EF0C
Lecture 1 —— Symbolic vs Numeric: relating computations in discrete and continuous structures
Speaker: Victor Selivanov (St. Petersburg State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Symbolic algorithms provide precise solutions (presented in a suitable form) and are widely used in computer algebra systems. Numeric algorithms provide approximate solutions and are widely used in numerical packages.
This talk is a short informal survey of some results on computable fields of real numbers and their applications to some computation problems in linear algebra and analysis (symmetric hyperbolic systems of PDEs with constant coefficients). Along with general computability (without any bound on computational resources) we discuss primitive recursive and polynomial-time computability. We trace how more efficient algorithms on fields of reals (which are symbolic algorithms on countable discrete structures) yield more efficient numeric algorithms on continuous structures (like the reals and other relevant metric spaces). From available competing models of computation on continuous structures we choose the model going back to Turing that arguably better corresponds to numeric algorithms (namely, to algorithms with arbitrary guaranteed precision). Our results suggest that adequate mathematical foundations for symbolic and numeric computations are provided by, respectively, computable model theory and computable analysis.
All results mentioned in the talk are joint with either Svetlana Selivanova or Pavel Alaev.
Bio: Professor Victor Lvovich Selivanov is an outstanding expert in Theory of Computation, Logic, Automata Theory, Computability in Analysis. He has got his PhD degree in 1979 with the thesis topic 'On computable numbering' and the DS/Habilitation in 1990 on 'Hierarchical Classification of Arithmetic Sets and Index Sets'.
Victor Lvovich previously worked at A.P. Ershov Institute of Informatics Systems (IIS), Siberian Branch of the Russian Academy of Sciences in Novosibirsk at Novosibirsk State University and Kazan Federal University. He has got multiple prestigious awards and grants on national and international levels.
Nowadays, Victor Selivanov is a professor of the Department of Mathematics and Computer Science at Saint Petersburg State University.
Lecture 2 —— Volume and proportionality of the moduli of Shtukas
Speaker: Zhiwei Yun (Massachusetts Institute of Technology)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The volume of a locally symmetric space is essentially a product of special values of zeta functions. More generally, Hirzebruch's proportionality theorem (extended by Mumford) tells us how to integrate any Chern class polynomial on a locally symmetric space.
We give an analog and extension of these results in the function field case where new phenomena show up. Locally symmetric spaces will be replaced by the moduli space of Drinfeld Shtukas with multiple legs, and special values of zeta functions will be replaced by a linear combination of their derivatives of various order. This is joint work with Tony Feng and Wei Zhang.
Bio: Yun received his Bachelor's degree from Peking University in 2004, and the PhD degree from Princeton University in 2009, under the supervision of Robert MacPherson. He had faculty appointments at Stanford and at Yale, before joining MIT as a Professor.
Yun's research is at the crossroads between algebraic geometry, number theory, and representation theory. He studies geometric structures aiming at solving problems in representation theory and number theory, especially those in the Langlands program.
Yun received the 2012 SASTRA Ramanujan Prize, the 2013 Packard Fellowship, 2018 New Horizons Prize, 2019 Gold Medal of the International Congress of Chinese Mathematics and 2020 Simons Investigatorship. He was an invited speaker at ICM 2018, and was selected to be Fellow of the AMS in 2019.
*********************
Lecture Series 72 —— March 13, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAEC61DEDB0F2B4B6086630973D789A869
Lecture 1 —— The Boltzmann equation for kinetic shear flow
Speaker: Renjun Duan (The Chinese University of Hong Kong)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The first part of the talk is to survey recent results on the kinetic shear flow governed by the nonlinear Boltzmann equation in both spatially homogeneous and inhomogeneous settings. In the second part, we focus on a specific problem on the 3D kinetic Couette flow in the diffusive limit.
Bio: Renjun Duan is currently a Professor in the Department of Mathematics at The Chinese University of Hong Kong (CUHK). He earned his PhD in Mathematics from the City University of Hong Kong in 2008. After graduation, he conducted postdoctoral research for two years at the Johann Radon Institute for Computational and Applied Mathematics (RICAM), part of the Austrian Academy of Sciences. In 2010, he joined CUHK, where he has been working ever since.
His research focuses on the analysis of partial differential equations (PDEs), with a particular emphasis on the Boltzmann equation in kinetic theory and its connections to fluid dynamic equations.
Lecture 2 —— Reconstruction of metric measure spaces and the spectral information on distances
Speaker: Eugene Stepanov (St. Petersburg Department of Steklov Mathematical Institute of RAS, University of Pisa)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The famous Gromov-Vershik metric measure space reconstruction theorem gives a way to reconstruct uniquely the metric measure space (up to a measure preserving isometry) by a suitable information on distances between randomly chosen points in the latter. The natural questions are then what can be reconstructed from the spectral information on random distance matrices. We will discuss some of these problems and their relationship to the multidimensional scaling, a widely used method in manifold learning.
Bio: Professor Eugene Stepanov is an outstanding expert in metric geometry and geometric measure theory with a broad range of applications, in particular in control, dynamical systems and calculus of variations. Having obtained the PhD. degree in 1999, by Scuola Normale Superiore, Pisa, Italy, he obtained the Doctor of Sciences (equivalent to Habilitation in France or Germany), from Institute of Information Transmission problems, Moscow, Russian Federation in 2006.
He has worked as a professor at the Department of Mathematical Physics of Saint Petersburg State University. Nowadays, he works at University of Pisa, combining this with a position at Saint Petersburg Department of Steklov Institute.
*********************
Lecture Series 71 —— January 9, 2025(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAF9FB16CCB18348769D016827C6B2C10C
Lecture 1 —— Some results on the steady solution for the incompressible Euler equation
Speaker: Daomin Cao (Academy of Mathematics and Systems Science, CAS)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: For the 2D incompressible Euler equation, there is a lot of results on the existence of vortex solutions, such as solutions with vorticity compactly supported or vorticity concentrated in several small sets. For the 3D case, the situation is much more difficult. A well-known solution is the vortex ring with small cross-section, traveling with a constant speed along its symmetric-axis, which was observed by Helmohtz in 1858. To get existence of solutions, people usually consider axi-symmetric case and a lot of axi-symmetric solutions have been established by different methods. But very little is known about the uniqueness. The stability of such vortex ring with small cross-section is a long-time open problem. In this talk the speaker will first talk about the uniqueness of such vortex ring. Then the speaker will introduce results on the nonlinear stability of such vortex ring. Lastly the speaker will talk about solutions with helical symmetry. The results presented in this talk are from joint papers with Guolin Qin, Weilin Yu, Weicheng Zhan and Changjun Zou.
Bio: Cao Daomin is currently a professor at the Academy of Mathematics and Systems Science, China Academy of Sciences. He received his doctorate from China Academy of Sciences in 1989. He is mainly engaged in the study of nonlinear partial differential equations and nonlinear variational problems. In recent years, he mainly studies the existence and stability of steady solutions of incompressible Euler equations. He is currently associated editor of“Acta Mathematicae Applicatae Sinica”and“Acta Mathematica Scientia”, and is a member of the editorial board of“Applied Analysis, Annales Academiae Scientiarum Fennicae, Mathematica”, etc.
Lecture 2 —— Potential Theory on graphs and embedding inequalities
Speaker: Pavel Mozolyako (St. Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract:
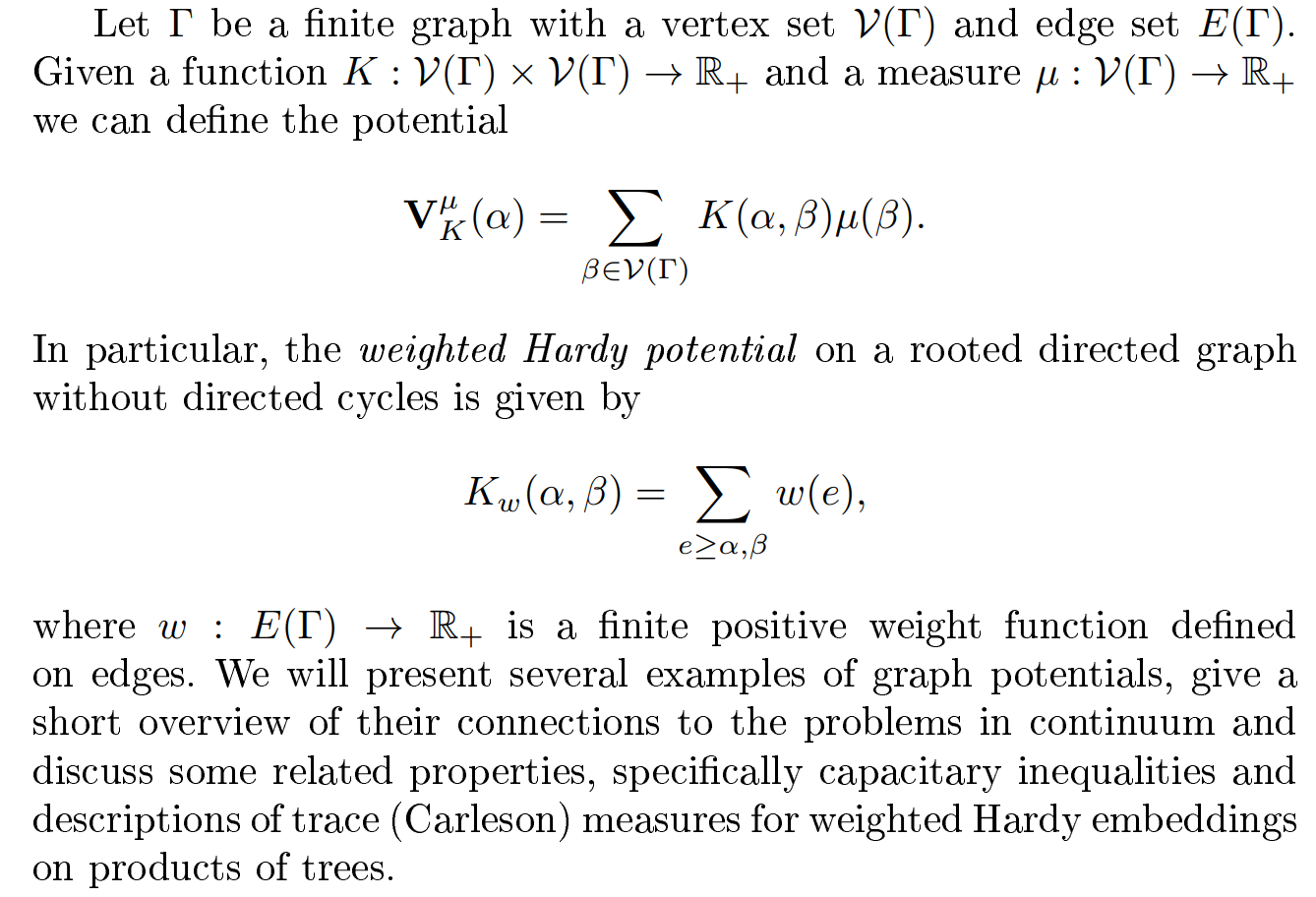
Bio: Dr. Pavel Mozolyako is a brilliant expert in Analysis. His research interests include:
- harmonic functions
- boundary behavior
- discrete models
- p-harmonic functions
- Dirichlet spaces
- growth classes
- wavelets.
Pavel Mozolyako has got his PhD degree in 2009, at St. Petersburg Department of Steklov Mathematical Institute of Russian Academy of Sciences and a year ago, has obtained the Doctor of Sciences/Habilitation degree. He was a postdoc at Norwegian University of Science and Technology, University of Bologna, and a visiting professor at Michigan State University before obtaining his current position at the Department of Mathematics and Computer Sciences.
*********************
Lecture Series 70 —— December 26, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA4698106E7CE74785B8E3CA1689DE23C5
Lecture 1 —— Gan-Gross-Prasad conjecture and its application in number theory
Speaker: Yifeng Liu (Zhejiang University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Gan-Gross-Prasad conjecture is one of the major conjectures in the area of automorphic forms, relating period integrals to L-values. In this talk, we will introduce this conjecture and survey its progress, mainly in the hermitian case, which has been fully solved. Then we will discuss its four applications in the Beilinson-Bloch-Kato conjecture, Iwasawa's main conjecture, subconvex bounds of L-values, and nonvanishing of L-values.
Bio: Yifeng Liu, chair professor at the Institute for Advanced Study in Mathematics, Zhejiang University. He graduated from Peking University in 2007, obtained PhD from Columbia University in 2012, under the supervision of Shouwu Zhang. He was then a C.L.E. Moore Instructor at MIT, an assistant professor at Northwestern University, an associate professor and a professor at Yale University, before returning to China in 2021. His main research interests include Algebraic Number Theory, Algebraic Geometry, and Automorphic Forms. He was awarded a SASTRA Ramanujan Prize in 2018.
Lecture 2 —— The de Rham cohomology of soft function algebras
Speaker: Igor Baskov (St. Petersburg Department of Steklov Mathematical Institute of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract:

The talk is based on the papers https://arxiv.org/abs/2208.11431, https://arxiv.org/abs/2304.04328 and https://arxiv.org/abs/2408.08689.
Bio: Igor Baskov is a brilliant young researcher, working in the areas of algebra and geometry. His research interests cover Deformation theory, Non-commutative geometry and algebraic de Rham cohomology. He graduated from Saint Petersburg State University and works at Saint Petersburg Department of Steklov Institute. Recently, he received the annual Steklov Institute prize for young scientists.
*********************
Lecture Series 69 —— December 12, 2024(21:00-23:00 Beijing time / 16:00-18:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA9086F216A35349A4AF063C593D433FD6
Lecture 1 —— On second eigenvalues of closed hyperbolic surfaces for large genus
Speaker: Yunhui Wu (Yau Mathematical Sciences Center, Tsinghua University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In this work we obtain optimal lower and upper bounds for second eigenvalues of closed hyperbolic surfaces for large genus. Moreover, we also study their asymptotic behaviors on random hyperbolic surfaces. This is a joint work with Yuxin He.
Bio: Yunhui Wu is a dual-appointed professor at the Yau Mathematical Sciences Center and Department of Mathematical Sciences of Tsinghua University. He obtained PhD degree from Brown University in 2012 and did his Postdoc at Rice University from 2012 to 2016. He then joined Tsinghua University in 2016. His research area is in Teichmüller theory of Riemann surfaces and Geometry.
Lecture 2 —— Concurrent normals problem for convex polytopes
Speaker: Gaiane Panina (St. Petersburg Department of Steklov Mathematical Institute of RAS)
Time: 22:00-23:00 Beijing time (17:00-18:00 St Petersburg time)
Abstract: This is a joint work with I. Nasonov.
It is conjectured since long that for any convex body $P\subset \mathbb{R}^n$ there exists a point in its interior which belongs to at least $2n$ normals from different points on the boundary of $P$. The conjecture is known to be true for $n=2,3$ (E. Heil, 1985) and $n=4$ (J. Pardon, 2012).
We treat the same problem for convex polytopes and prove that each simple polytope in $\mathbb{R}^3$ has a point in its interior with 10 normals to the boundary. This is an exact bound: there exists a tetrahedron with at most 10 normals from a point in its interior. The proof is based on Morse–Cerf theory adjusted for polytopes.
Bio: Professor Gaiane Panina is an outstanding expert in Geometry and Topology.
Prof. Panina was born in Leningrad, now Saint Petersburg (she is a granddaughter of Acad. Victor Ambartsumian, one of the most known Soviet Astronomers). She graduated from Leningrad State University where she has got her PhD thesis in 1989.
In 2007, she has got her habilitation (Doctor of Science degree) with the thesis named 'Virtual polytopes'. Her research interests cover a broad area including but not are limited to: combinatorial geometry, polyhedral combinatorics, polygons, spaces of moduli, discrete Morse theory, universality, hinged mechanisms.
Now Prof. Panina works at Steklov Mathematical Institute of Russian Academy of Sciences, combining this with teaching at Saint Petersburg State University (Department of Mathematics and Computer Science) and PDMI Physics and Mathematics Club.
*********************
Lecture Series 68 —— November 28, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAEE6EE3DCAB384FE79258F879CF21499C
Lecture 1 —— Some results on the constrained variational problem
Speaker: Wenming Zou (Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: This survey talk mainly involves two parts: The normalized solution and the stability of inequality. The first part will focus on the existence of solutions to some Schrodinger systems or equation satisfying the normalization constraint, including the normalized solutions to the BEC-type systems with a wider range of parameters and the existence of normalized solutions of nonlinear Schrodinger equations with combined nonlinearities in large bounded domains. The second part of the talk is concerned with the quantitative stability for several inequality, such as Sobolev trace inequality and CKN-inequality.
Bio: Wenming Zou is a professor at the Math Department, Tsinghua University. He obtained PhD in 1998 from the Institute of Mathematics, Chinese Academy of Sciences; Conducted postdoc research at Stockholm University from 1998 to 1999; Visiting assistant professor and lecturer at the University of California, Irvine from 2001 to 2004; Published over 170 research articles and two monographs in Springer-NY, including journals as Math Ann; ARMA; Adv Math; JMPA; ComPDE; AIHP; TransAMS; JFA; SIAM-JMA; Ann. Scuola Normale Pisa; CVPDE; etc. MathSciNet shows that the works have been cited over 4200 times, sparking and inspiring a series of subsequent research by others.
Lecture 2 —— Statistical estimation theory using perturbed optimization
Speaker: Vladimir Spokoiny (Weierstrass Institute for Applied Analysis and Stochastics)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The talk discusses estimation problem for complex high-dimensional models like Deep Neuronal Networks (DNN). The approach reduces the original problem to perturbed optimization. Under some mild conditions, we establish finite sample expansions for the loss of estimation and for the excess risk. This enables us to obtain sharp nonasymptotic risk bounds in terms of the so called efficient dimension of the problem. The results are specified to the case of nonlinear regression and DNN training.
Bio: Professor Vladimir Spokoiny is a top-level expert in Machine Learning, Statistics and Applied Analysis. Having graduated in 1981 from the Department of Technical Cybernetics at Moscow Institute of Railway Engineering, he obtained his PhD degree at Moscow State University under supervision of Prof. M.B. Maljutov and Prof. A.N. Shiryaev.
Prof. Spokoiny worked at Moscow State University, University Paris-Sud and University of Wurtzburg. Now he heads a research group at Weierstrass Institute combining this with a professor position at Humboldt University being is associated editor of Annals of Statistics and Statistics and Decisions journals.
His research interests are related with:
- adaptive nonparametric smoothing and hypothesis testing
- high dimensional data analysis
- statistical methods in finance
- image analysis, applications to medicine
- classification
- nonlinear time series.
*********************
Lecture Series 67 —— November 14, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA9FAACCB1B9E844DA891C9A854FA49AB7
Lecture 1 —— Commutator type and Levi type of a system of CR vector fields
Speaker: Wanke Yin (Wuhan University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Finite type conditions arise naturally during the study of weakly pseudoconvex hypersurfaces in $\mathbb{C}^n$, which are defined to measure to degeneracy of the Levi form. Let $M$ be a pseudoconvex hypersurface in $\mathbb{C}^n$, $p\in M$, and let $B$ be a subbundle of the CR tangent bundle $T^{(1,0)}M$. The commutator type $t(B,p)$ measures the number of commutators of the sections of $B$ and their conjugates needed to generate the contact tangent vector at $p$. The Levi type $c(B,p)$ is concerned with differentiating the Levi form along the sections of $B$ and their conjugates. It is believed that these two types are the same, which is known as the generalized D'Angelo Conjecture. In this talk, I shall talk about the recent progress on this conjecture, which is based on the joint works with X. Huang and P. Yuan.
Bio: Wanke Yin is a professor at Wuhan University. He received his PhD degree from Wuhan University in 2008, and has been working there ever since. Professor Yin's primary research interest is Several Complex Variables and related fields.
Lecture 2 —— Real-Valued Spacetime Dimensions, Black Hole Dualities, and the Riemann Zeta Function
Speaker: Irina Aref'eva (Steklov Mathematical Institute of Russian Academy of Sciences)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Multidimensional spaces are widely used in mathematics and physics. It is well known that we live in a 3+1 spacetime. Models in lower-dimensional spacetimes are often employed as toy models to explore specific processes under simplified conditions.
In physics, models in higher-dimensional spacetimes are not merely mathematical generalizations of our observed reality; they can encode essential physical information. In this context, it is worth mentioning the Kaluza-Klein theory, superstring theory, and others. A special role is played by five-dimensional models, known as holographic models, in which the fifth coordinate serves as an auxiliary dimension representing the energy scale.
This talk will also address the emergence of negative dimensions, which appear when attempting to describe black hole thermodynamics using quantum statistical models. The origin of this phenomenon lies in the violation of the third law of thermodynamics (in Planck’s formulation) by the Schwarzschild black hole. To assign mathematical meaning to geometrical objects in negative dimensions, we use analytical continuation and properties of the Riemann zeta function.
The talk is based on:
I. Y. Aref'eva et al,“Holographic QCD running coupling for light quarks in strong magnetic field,”Phys. Rev. D 110, 086021 (2024)
I. Y. Aref'eva and I. V. Volovich,“Bose gas modeling of the Schwarzschild black hole thermodynamics,”Theor. Math. Phys. 218, 192-204 (2024)
I. Aref'eva and I.Volovich,“Violation of the Third Law of Thermodynamics by Black Holes, Riemann Zeta Function and Bose Gas in Negative Dimensions,”Eur. Phys. J. Plus 139, 300 (2024)
Bio: Irina Yaroslavna Arefyeva is an outstanding Russian mathematician and theoretical physicist, Doctor of Physical and Mathematical Sciences, Professor, and Corresponding Member of the Russian Academy of Sciences since 2022.
She graduated from the Physics Department of Leningrad State University in 1970 and completed her postgraduate studies in this department. In 1973, she defended her PhD thesis on "Scattering Theory in Constructive Quantum Field Theory". In 1977, the results of her thesis were appreciated by the USSR Academy of Sciences - she received the Gold Medal for Young Scientists.
In 1982, Irina Yaroslavna defended her doctoral dissertation on the topic "On renormalizability in the framework of the 1/N expansion of nonlinear sigma models".
Starting from 1970, Prof. Aref'eva is working at the Steklov Mathematical Institute, first in Leningrad (Saint Petersburg), then in Moscow. Now, she has a position of Chief Researcher of the Department of Theoretical Physics. Prof Aref'eva is a member of the editorial board of the journals "Journal of Physics A, Mathematical and General", Institute of Physics Publishing, Bristol, UK, (2000-2006) and "Theoretical and Mathematical Physics" (since 2008).
She was one of the first to put forward the hypothesis about the possibility of black hole production in accelerators within the framework of TeV gravity, showed that a model that is not renormalizable according to the standard perturbation theory turns out to be renormalizable in the inverse number of field components (the so-called 1/N-expansion), solved the problem of constructing a theory free of ultraviolet and infrared divergences for an essentially nonlinear three-dimensional n-field. Her work on the contour approach in gauge field theory is widely used in quantum chromodynamics; obtained estimates of the formation time of quark-gluon plasma and the total multiplicity of particles formed depending on the properties of colliding heavy ions within the framework of the holographic approach.
Prof. Aref'eva's areas include: Renormalization theory, Superstring theory, D-branes, duality between gauge theories and superstring models.
*********************
Lecture Series 66 —— October 31, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AABCAC4B4FB4294DCEAE32637CBB69A429
Lecture 1 —— Polynomial formulations as a barrier for reduction-based hardness proofs
Speaker: Alexander Kulikov (Saint Petersburg State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The Strong Exponential Time Hypothesis (SETH) postulates that SAT cannot be solved in less than 2^n steps. In recent years, many SETH-based lower bounds have been proven: for example, n^2 for Edit Distance, 2^n for Hitting Set, 2^{treewidth} for Independent Set. One may speculate that SETH can explain many other current algorithmic barriers. In the talk, we'll show that, for many problems, an SETH lower bound would imply new circuit lower bounds.
Bio: Professor Alexander S. Kulikov has graduated from Saint Petersburg State University at 2005, has got his PhD degree in 2009 and Doctor of Science degree at 2017 (under supervision of Prof. A Hircsh, the topic of his thesis was Complexity of Explicitly Defined Boolean Functions).
Prof. Kulikov combines a full-professor position at the Faculty of Mathematics and Computer Science at Saint Petersburg State University with that of Senior Research Fellow of Steklov Institute. Besides, he is the founder and a board member of the Computer Science Center at Steklov Institute.
Prof. Kulikov is a top-level expert in Logics, Algorithms, and Complexity Theory. He has got 44 research papers and one book in these areas. Moreover, he has got some prestigious prized, including Best Young Researcher Award by Steklov Institute of Mathematics at St. Petersburg, Russian Academy of Science and Scopus Award Russia by Elsevier.
Lecture 2 —— The Picard group of moduli spaces of K3 surfaces and their compactifications
Speaker: Zhiyuan Li (Shanghai Center for Mathematical Sciences, Fudan University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The study of Picard group of moduli spaces is an important question in moduli theory. Since from Mumford's famous paper “Picard groups of moduli problems”, algebraic geometers have investigated the Picard group of moduli space of smooth curves and its compactifications. It has been shown by Harer and Arbarello-Cornalba that M_g has Picard number one while its Deligne-Mumford compactification \overline{M}_g has Picard number 2+[g/2]. In this talk, I will survey the recent progress for moduli spaces of projective K3 surfaces and their compactifications. We will give a complete description of their Picard groups.
Bio: Zhiyuan Li is a dual-appointed professor at Shanghai Center for Mathematical Sciences and School of Mathematical Sciences of Fudan University. He was selected for the National High level Talent Program. He graduated from University of Science and Technology of China in 2004, and received the PhD degree from Rice University in 2012. He has worked as a postdoctoral fellow at Stanford University and University of Bonn.
Professor Zhiyuan Li is mainly engaged in the research of algebraic geometry and related fields and has made a series of achievements in moduli space in algebraic geometry and hyper-Kähler Geometry. He has solved long-term unsolved public problems such as the Noether-Lefschetz conjecture on the moduli space of K3 surfaces and the special Hodge conjecture on orthogonal Shimura varieties, and published a number of papers in important international journals such as Inventions and Duke Mathematical Journal.
*********************
Lecture Series 65 —— June 6, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Lecture 1 —— Post-groups, post-groupoids and the Yang-Baxter equation
Speaker: Yunhe Sheng (Jilin University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We introduce the notion of post-groups, which are the underlying structures of Rota-Baxter operators on groups. The differentiation of post-Lie groups gives post-Lie algebras. Post-groups are also related to braces and Lie-Butcher groups, and give rise to set-theoretical solutions of Yang-Baxter equations. We further introduce the notion of post-groupoids, whose differentiations are post-Lie algebroids. We show that post-groupoids give quiver-theoretical solutions of the Yang-Baxter equation on the underlying quiver of the subadjacent groupoids.
The talk is based on the joint work with Chengming Bai, Li Guo, Rong Tang and Chenchang Zhu.
Bio: Yunhe Sheng is a Professor in Jilin University. He received his Ph. D degree from Peking University in 2009, and then spent one year in Goettingen University as a post-doctor. His research interests are Poisson geometry, higher Lie theory and mathematical physics.
Lecture 2 —— Some classification problems and orbits of algebraic groups
Speaker: Vladimir L. Popov (Steklov Mathematical Institute of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: There are many examples where the problem of classifying algebraic objects of a certain type is reformulated as that of classifying orbits of some algebraic group action. The talk is aimed to discuss the decidability of the equivalence problem for two objects of the considered type in such cases.
Bio: Professor Vladimir Leonidovich Popov is a principal researcher at the Steklov Mathematical Institute of Russian Academy of Sciences in Moscow and also a professor at Department of Applied Mathematics of MIEM-HSE (part time). He graduated from Mathematics and Mechanics Faculty of Moscow State University Lomonosov (Department of High Algebra) in 1969 and got his PhD degree (Candidate of Physics and Mathematics) in 1972. He obtained the Doctor of Physics and Mathematics in 1984. Professor Vladimir Popov was elected as a Corresponding Member of the Russian Academy of Sciences in October 2016.
His research interests are algebraic groups, Lie groups, Lie algebras and their representations; algebraic geometry; algebraic transformation groups; invariant theory; automorphism groups of algebraic varieties; discrete reflection groups.
*********************
Lecture Series 64 —— May 23, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time)
Lecture 1 —— Contact +1 surgeries and vanishing of contact homology
Speaker: Zhengyi Zhou (Morningside Center of Mathematics, Academy of Mathematics and Systems Science)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: From a surgical perspective, every contact manifold can be obtained from applying both isotropic and coisotropic surgeries on the standard contact sphere. Vanishing of contact homology, i.e. the incarnation of overtwistedness in symplectic field theory, can only arise from coisotropic surgeries, in particular, contact +1 surgeries. In this talk, I will explain situations of contact +1 surgeries yielding vanishing of contact homology both in dimension 3 and higher dimensions. In particular, I will explain contact +1 surgeries along any torus knots with maximal Thurston-Bennequin number produce tight contact manifolds with vanishing contact homology. This is partially joint with Youlin Li.
Bio: Zhengyi Zhou received his PhD degree form UC Berkeley in 2018. After that, he spent three years at IAS princeton as a postdoc. Starting from 2021, he is an associate professor at the Morningside Center of Mathematics, Academy of Mathematics and Systems Science. His research interest lies in symplectic and contact topology.
Lecture 2 —— The Chern–Dold character and the Milnor–Hirzebruch problem
Speaker: Victor Buchstaber (Steklov Mathematical Institute, Moscow Steklov International Mathematical Center, MSU)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The talk is devoted to problems and results related to the Chern numbers of complex, algebraic and toric manifolds.
Our goal is to present a recent result of Buchstaber and Veselov: The exponential generating series of complex cobordism classes of theta divisors of principally polarized Abelian varieties realizes the exponential of the formal group of geometric cobordisms.
This result is based on Buchstaber's construction (1970) of the Chern–Dold character in the theory of complex cobordism.
We will discuss applications of this result to well-known problems in algebraic topology and algebraic geometry, including the hitherto open Milnor–Hirzebruch problem (1958) on Chern numbers of irreducible smooth algebraic manifolds.
Bio: Professor Victor Buchstaber was born 01 April 1943. In 1966 he graduated from Moscow Lomonosov State University (MSU). He is a Doctor of Physical and Mathematical Sciences, Corresponding Member of the Russian Academy of Sciences, Professor of the Department of Higher Geometry and Topology at the Moscow State University, Chief Researcher of the Department of Geometry and Topology at the Steklov Mathematical Institute RAS, Vice-President of the Moscow Mathematical Society, Corresponding Fellow of the Royal Society of Edinburgh (UK), Emiritus Professor at the University of Manchester (UK), Honorary Doctor at the Voronezh State University (Russia).
Areas of his scientific interests: algebraic topology, functional equations, theory of Abelian functions, mathematical physics, applied mathematics.
He is author of more than 300 scientific publications. Monograph "Toric Topology" by V.M. Buchstaber and T.E. Panov (AMS, 2015) became world famous.
Victor Buchstaber has a large scientific school. More than 30 dissertations were defended under his scientific supervision. Many of his students became famous scientists and received worldwide recognition.
*********************
Lecture Series 63 —— May 16, 2024 (20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA7E55187970EA4D21BDEBC05250CD3B75
Lecture 1 —— On the hydrostatic approximation of the 3D Boussinesq equations of damped wave type
Speaker: Weixi Li (Wuhan University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We study the hydrostatic approximation for the three-dimensional Boussinesq equations of damped wave type. This is a mixed degenerate system coupled by parabolic and hyperbolic equations. Compared with the purely hyperbolic hydrostatic Navier-Stokes equations, the parabolic equation for temperature will lead to an extra loss of derivatives. In the setting of Gevrey space with index 7/4, we prove the local well-posedness and the corresponding hydrostatic limit for the 3D Boussinesq equations of damped wave type.
Bio: Professor Weixi Li received his bachelor degree and PhD in 2003 and 2008, respectively, at Wuhan University. Li was appointed to Lecturer in 2008 and then promoted to Associate Professor in 2012 at Wuhan University, and since 2014 he is a full Professor at the same university. Li was postdoc fellows at universities of Paris VI, Lund, Nantes and Bologna from 2009 – 2012. His research interest lies in the microlocal analysis and its application in kinetic and fluid mechanics equations.
Lecture 2 —— On equations of integrable billiard tables
Speaker: Andrey Mironov (Sobolev Institute of Mathematics)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We will consider several questions related to the integrability of mathematical billiards. In particular, we consider a method to find differential equations for functions which define tables, such that associated billiard systems admit a local first integral. The talk is based on a joint paper V. Dragovic, A. E. Mironov, Acta Mathematica Sinica, 2024.
Bio: Andrey Mironov is the Acting Director of the Sobolev Institute of Mathematics, Novosibirsk, Russia. He is a Corresponding member of the Russian Academy of Sciences. Prof. Mironov’s research focuses on integrable systems, geometry and mathematical physics.
*********************
Lecture Series 62 —— April 25, 2024 (20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Password: U0BP
Lecture 1 —— Estimates of the proximity of successive convolutions of the probability distributions on the convex sets and in the Prokhorov distance
Speaker: Zaitsev Andrei Yurievich(St.-Petersburg Department of Steklov Mathematical Institute)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Let $X_1,\dots, X_n,\dots$ be independent identically distributed $d$-dimensional random vectors with common distribution $F$. Then $S_n = X_1+\dots+X_n$ has distribution $F^n$ (degree is understood in the sense of convolutions). Let $$\rho(F,G) = \sup_A |F\{A\} - G\{A\}|,$$ where the supremum is taken over all convex subsets of $\mathbb R^d$. Basic result is as follows. For any nontrivial distribution $F$ there is $c(F)$ such that $$\rho(F^n, F^{n+1})\leq \frac{c(F)}{\sqrt n}$$ for any natural $n$. The distribution $F$ is considered trivial if it is concentrated on a hyperplane that does not contain the origin. Clearly, for such $F$ $$\rho(F^n, F^{n+1}) = 1.$$ A similar result is obtained for the Prokhorov distance between distributions normalized by the square root of $n$.
Bio: Zaitsev Andrei works at the St.-Petersburg Department of Steklov Mathematical Institute (1978–now);the frea of interest now - Probability Theory, Probability Limit Theorems, Invariance Principles, Infinitely Divisible Distributions, Strong Approximation, Kernel density Estimators, Concentration Functions.
Lecture 2 —— Bohr chaoticity and Khintchin conjecture
Speaker: Aihua Fan (Picardie University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The Sarnak conjecture, which concerns with the Birkhoff averages weighted by the Möbius sequence, asserts that all zero entropy systems are orthogonal to the Möbius sequence. Which systems are orthogonal to none of non-trivial weights? We define such systems as Bohr chaotic systems. The Bohr chaoticity is a complexity of the system and is a topological invariant; it implies the positivity of entropy. However, the positivity of entropy doesn’t imply the Bohr chaoticity. We prove that a system (X, T) admitting a horseshoe (i.e a susbsytem of some power of T is conjugate to a full shift) is Bohr chaotic. Thus the usual nice systems of positive entropy are Bohr chaotic. But systems having few ergodic measures are not Bohr chaotic. Another class of systems which are proved to be Bohr chaotic are the algebraic principal systems. These are joint works with Shilei FAN (Wuhan), Valery RYZHYKOV (Moscou), Klaus SCHMIDT (Vienna), Weixiao SHEN (Shanghai) and Evgeny VERBITSKIY (Leiden). Also I would like to talk about Khintchin’s conjecture, a related problem in a setting of actions of mutiplicative semigroups of integers (more generally, actions of surjective endomorphisms of a compact Abelian group). But there is more questions than results for this topic.
Bio: Aihua FAN is a professor from Picardie University, France and a guest professor from Wuhan University, China. He is interested in Ergodic theory and Dynamical systems, Fourier Analysis, Probability and Fractal geometry. In particular, he contributed to the ergodic theorems and their generalizations, the dynamical Diophantine approximation, the p-adic dynamics, the dimension theory of measures, the multifractal analysis and thermodynamical formalism, the theory of multiplicative chaos (Levy chaos, Mandelbrot cascades, percolation on trees, Dvoretzky covering), the Riesz products and lacunary trigonometric series, Fuglede spectral conjecture.
*********************
Lecture Series 61 —— April 11, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA931BE5582F5640D68E122F37BB4E4E29
Valid Until: 2025-04-30 09:11
Lecture 1 —— Jacobian determinants for nonlinear gradient of planar $\infty$-harmonic functions and applications
Speaker: Yuan Zhou(Beijing normal university)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In dimension 2, we introduce a distributional Jacobian determinant for the nonlinear complex gradient $V_\beta(Dv)$ of a function $v\in W^{1,2 }_\loc$ with $\beta |Dv|^{1+\beta}\in W^{1,2}_\loc$, where $\beta>-1$. This is new when $\beta\ne0$. Given any planar $\infty$-harmonic function $u$, we show that such distributional Jacobian determinant $\det DV_\beta(Du)$ is a nonnegative Radon measure with some quantitative local lower and upper bounds. Denoting by $u_p$ the $p$-harmonic function having the same nonconstant boundary condition as $u$, we show that $\det DV_\beta(Du_p) \to \det DV_\beta(Du)$ as $p\to\infty$ in the weak-$\star$ sense in the space of Radon measure. Recall that $V_\beta(Du_p)$ is always quasiregular mappings, but $V_\beta(Du)$ is not in general.
Bio: Yuan Zhou is a professor from Beijing normal university. He is interested in function spaces, quasiconformal mappings, nonsmooth analysis in metric measure space, quasilinear equations, semilinear equations and also infinity Laplacian and Aronsson equations. In particular, he contributed to the second order regularity of planar infinity harmonic functions, the coincidence of distance and differential structures of metric spaces, and quasiconformality of Triebel-Lizorkin spaces.
Lecture 2 —— Solid mechanics models in ophthalmology
Speaker: Eva Voronkova(Saint Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Biomechanics is the application of mechanics to understand better the structure, properties, and function of biological systems. The human eye is a remarkably complex structure with biomechanics involved in many of its functions. Solid mechanics-based models have been used in recent years as tools to describe, for example, the stress-strain state of the eye shell under the encircling band; to build a biomechanical model of the choroidal detachment, to depict the different mechanical aspects of the development of glaucomatous atrophy of the optic nerve fibres and the behaviour of Lamina Cribrosa - a circular or closed to a circular plate, where the optic nerve fibres pass. The talk will review some of these models, with a focus on the mathematical models for various types of tonometers and differences in the intraocular pressure readings (IOPR) before and after vision correction surgeries.
Bio: Dr Voronkova graduated from the Faculty of Mathemaics and Mechanics in 1999.She has an experience of working at KTH, Stockholm, and Munchen Technical University in Germany. Nowadays Dr. Voronkova works as an associate professor of the Department of Mathematical Modeling of Energy Systems of Saint Petersburg State University. She is a brilliant expert in Asymptotic and numerical methods in the theory of plates and shells, Nonclassical theories of plates and shells, Mathematical modeling in biomechanics, and Modeling of soft biological tissues. Besides, she is a laureate of several prestigious grants and awards on national and international level.
*********************
Lecture Series 60 —— March 28, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAAE6B05EEFDE842E69D3E77556A0378E4
Valid Until: 2025-04-30 10:17
Lecture 1 —— Compactness of asymptotically hyperbolic Einstein manifolds in dimension 4 and applications
Speaker: Yuxin Ge (University of toulouse 3)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Given a closed riemannian manfiold of dimension 3 (M3,[h]), when will we fill in an asymp totically hyperbolic Einstein manifold of dimension 4 (X4,g+) such that r2g+|M = h on the boundary M = ∂X for some defining function r on X4? This problem is motivated by the correspondance AdS/CFT in quantum gravity proposed by Maldacena in 1998 et comes also from the study of the structure of asymptotically hyperbolic Einstein manifolds.
In this talk, I discuss the compactness issue of asymptotically hyperbolic Einstein manifolds in dimension 4, that is, how the compactness on conformal infinity leads to the compactness of the compactification of such manifolds under the suitable conditions on the topology and on some conformal invariants. As application, I discuss the uniqueness problem and non-existence result. It is based on the works with Alice Chang.
Bio: Yuxin Ge is a professor at University of toulouse 3. He defended his doctoral thesis at l'Ecole normale superieure de Cachan in 1997. His research mainly focuses on geometric analysis.
Lecture 2 —— Birational geometry of del Pezzo surfaces
Speaker: Constantin Shramov (Mathematical Institute of RAS; HSE)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A del Pezzo surface is a smooth projective surface with ample anticanonical divisor. Over an algebraically closed field, any surface like this is rational. However, without this assumption del
Pezzo surfaces exhibit very interesting birational properties. I will survey some old and new results about birational geometry of del Pezzo surfaces over arbitrary fields, mostly focusing on Severi–Brauer surfaces, quadrics, and del Pezzo surfaces of degree 4.
Bio: Professor Constantin Shramov obtained a Ph.D. degree in Mathematics from Moscow State University in 2007. He became researcher at Steklov Mathematical Institute in 2008. His research focuses on algebraic geometry.
********
Lecture Series 59 —— March 14, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA1E3D6434BAE046D8A17BC6CBF7D4AAA8
Valid Until: 2025-04-30 10:16
Lecture 1 —— Andronov School of Nonlinear Oscillations
Speaker: Olga Pochinka (Higher School of Economics)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Andronov's school began to take shape in 1931, when Alexander Alexandrovich himself, together with his wife E.A. Leontovich, moved from Moscow to Nizhny Novgorod.
Bio: Professor Olga Pochinka is currently the head of the Laboratory of Dynamic Systems and Applications of the Higher School of Economics, Russia, Nizhny Novgorod. She obtained Doctor of Physical and Mathematical Sciences, specialty differential equations since 2011, and became a full professor since 2023. Her research interests lie in Qualitative theory of dynamical systems, and has published more than 200 publications.
Lecture 2 —— Geometric interpretation for Navier-Stokes equations
Speaker: Shizan Fang (University of Burgundy)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: To velocity associated to Navier-Stokes equations, we try to give a geometric interpretation of relations between vorticity, helicity and strain tensor.
Bio: Shizan Fang obtained a Bachelor's degree from Wuhan University in 1985 and a Ph.D. degree in Mathematics from University of Paris VI in 1990.
******
Lecture Series 58 —— February 29, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Lecture 1 —— Hodge-Riemann relations for Schur classes
Speaker: Weizhe Zheng (Academy of Mathematics and Systems Science, CAS)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The hard Lefschetz theorem and the Hodge-Riemann bilinear relations for ample line bundles are important consequences of Hodge theory on projective varieties. I will give an overview of extensions of these properties to Chern and Schur classes of ample vector bundles.
Bio: Weizhe Zheng is a professor at the Academy of Mathematics and Systems Science, Chinese Academy of Sciences. He defended his doctoral thesis at l'Université Paris XI in 2007. His research mainly focuses on algebraic geometry and cohomology theories.
Lecture 2 —— Contou-Carrere symbols and Riemann-Roch theorems
Speaker: Denis Osipov (Steklov Mathematical Institute)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The Contou-Carrere symbol was introduced by C. Contou-Carrere and P. Deligne. This symbol generalizes the residue of differential form and the tame symbol at a point on an algebraic curve, and can be considered as the deformation of the tame symbol. By two invertible elements of the algebra of Laurent series over a commutative ring this symbol defines an invertible element of the base ring. The Contou-Carrere symbol is connected with the class field theory for an algebraic curve over a finite field, and it satisfies the reciprocity laws. In my talk I will speak about these classical results and also about my recent results on the connection of the Contou-Carrere symbol with the Grothendieck-Riemann-Roch theorem for the family of projective curves. This connection is via the central extension of the group that is the semidirect product of the group of invertible elements of the algebra of Laurent series over a ring and the group of continuous automorphisms of this algebra.
Bio: Denis Vasilyevich Osipov is a brilliant expert in algebraic and arithmetic geometry, algebraic number theory, multidimensional adeles and multidimensional local fields, 2-categories and their applications to multidimensional adeles and arithmetic, integrable systems.
He graduated from the Faculty of Mechanics and Mathematics of Moscow State University in 1996 and got his PhD there in 1999 and the DS degree (habilitation) in 2013. Later on, in 2018, Prof. Osipov has got the honorable degree of Professor of Russian Academy of Science.
******
Lecture Series 57 —— January 18, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AAA3DF6541B79A4F8CBD632A51D18CD1F4
Valid Until: 2025-02-18 10:43
Lecture 1 —— A geometric perspective on the compressible Euler equations
Speaker: Pin Yu (Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: I will provide an overview of the latest developments in the study of singularity formation and resolution in compressible Euler equations, with a specific focus on the Lorentzian geometry of the acoustical metrics.
Bio: Pin Yu is a professor at Tsinghua University. He received his PhD from Princeton University in 2010. His research focuses on nonlinear wave equations.
Lecture 2 —— Bourgain—Brezis inequalities and related topics
Speaker: Dmitriy Stolyarov (Saint Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Bourgain—Brezis inequalities is an informal name for a class of estimates that resemble the Sobolev embedding theorem and include the norms in the limit spaces L_1 or L_\infty. Those specific norms, one the one hand, make the inequalities harder to prove, and on the other hand, link them to problems in geometric measure theory. The phenomenon may be traced back to the work of Gagliardo and Nirenberg in late 50-s. It got an intensive development in the 2000-s motivated by a fresh insight by Bourgain and Brezis. Nowadays, the field gets its inspiration from connections to geometric measure theory. I will survey the field, draw the said connections, and, if time permits, outline the recent ``probabilistic’’ approach suggested by Ayoush, Wojciechowski, and me.
Bio: Dmitry Stolyarov is an outstanding expert in Functional Analysis. His research interests cover, in particular, Fourier Analysis, Bellman Functions, Banach Space Theory, and Regularized Traces. Dmitry graduated from the Faculty of Mathematics and Mechanics of Saint Petersburg State University at 2011. Three years later, he defended his PhD thesis devoted to Fourier Analysis and Embedding Theorems under supervision of Prof. S.V. Kislyakov. Now he works at the Faculty of Mathematics and Computer Science. Dmitry is a laureate of several prestigious awards and grants at national and international levels.
******
Lecture Series 56 —— January 4, 2024(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/6635F43357C55A250F7FD461806C16DC
Valid Until: 2025-02-01 23:59
Lecture 1 —— Multiple SLEs and Dyson Brownian motion
Speaker: Hao Wu (Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Multiple SLEs come naturally as the scaling limit of multiple interfaces in 2-dimensional statistical physics models. Dyson Brownian motion usually describes the movement of trajectory of independent Brownian motions under mutual repulsion. In this talk, we will describe the connection between multiple SLE and Dyson Brownian motion. It turns out that, under proper time-parameterization, and conditioning on a rare event, the driving function of multiple SLEs becomes Dyson Brownian motion. Using such a connection, we may translate estimates on Dyson Brownian motion to estimates on multiple SLEs.
Bio: Hao Wu obtained her bachelor’s degree from Tsinghua University in 2009 and obtained her PhD from University of Paris-sud in 2013. She was a C.L.E. Moore instructor at MIT from 2013 to 2015 and was a postdoc researcher at Geneva University from 2015 to 2017. In 2017, she came back to Tsinghua University as a professor.
Hao Wu works on statistical physical models such as stochastic process Schramm-Loewner Evolution, Gaussian free field and Ising model. Hao Wu’s series of works find the scaling limits of a general class of boundary-to-boundary connection probabilities and multiple interfaces in the 2-dimensional critical lattice models. They verified predictions from the physics literature and provided evidence of the expected conformal field theory of these models.
Lecture 2 —— Generative Flow Networks as Entropy-Regularized Reinforcement learning
Speaker: Alexey Naumov (National Research University Higher School of Economics)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The recently proposed generative flow networks (GFlowNets) are a method of training a policy to sample compositional discrete objects with probabilities proportional to a given reward via a sequence of actions. GFlowNets exploit the sequential nature of the problem, drawing parallels with reinforcement learning (RL). Our work extends the connection between RL and GFlowNets to a general case. We demonstrate how the task of learning a generative flow network can be efficiently redefined as an entropy-regularized RL problem with a specific reward and regularizer structure. Furthermore, we illustrate the practical efficiency of this reformulation by applying standard soft RL algorithms to GFlowNet training across several probabilistic modeling tasks. Contrary to previously reported results, we show that entropic RL approaches can be competitive against established GFlowNet training methods. This perspective opens a direct path for integrating reinforcement learning principles into the realm of generative flow networks. The talk is based on the joint work with Nikita Morozov, Daniil Tiapkin and Dmitry Vetrov.
Bio: Alexey Naumov is a professor and a head of the International laboratory of stochastic algorithms and high-dimensional inference of the National Research University Higher School of Economics. He graduated from the Lomonosov Moscow State University (MSU) in 2010, received a PhD degree in mathematics from Bielefeld University in 2013, candidate of science in mathematics and physics from MSU in 2013 and doctor of computer science degree in 2022. Alexey is the world-famous specialist in the areas of high-dimensional probability and mathematics of data science. His research interests span a broad range of problems extending from fundamental, theoretical questions in probability to applications in various branches of data science. Alexey Naumov is an author of more than 30 papers in leading journals and conferences of the field including Probability Theory and Related Fields, Bernoulli, Statistics and Computing, COLT, ICML and NeurIPS.
******
Lecture Series 55 —— December 21, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn/link/AA60505DD12D6F4DD3AC81F88F9974E7A8
Valid Until: 2025-03-30 09:42
Lecture 1 —— Infinitesimal extension of adjoint forms in Kähler setting and log injectivity theorem
Speaker: Junyan Cao (Université Côte d’Azur)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, we will first establish a very general ddbar-lemma, together with a decomposition theorem for currents with values in a (singular) Hermitian line bundle. As a corollary, we will establish the Kähler version of an injectivity theorem due to O. Fujino in the projective case, as well as some results concerning the invariance of the plurigenera conjecture in the Kähler setting. This work is a collaboration with Mihai Paun.
Bio: Junyan Cao works in the domain of complex geometry. After obtaining his PhD degree in Grenoble in 2013, he was a Maître de conférence at Sorbonne University and became a professor at Université Côte d’Azur in 2019.
Lecture 2 —— Minimal triangulations of manifolds like projective planes, and their symmetry groups
Speaker: Alexander Gaifullin (Steklov Mathematical Institute of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A theorem by Brehm and Kühnel (1987) says that a d-dimensional combinatorial manifold K (without boundary) with n vertices is PL homeomorphic to the sphere, provided that n is less than 3d/2+3. Moreover, if n is equal to 3d/2+3, then K is PL homeomorphic to either the sphere or a manifold like a projective plane, which exist in dimensions 2, 4, 8, and 16 only. There exists a 6-vertex triangulation of the real projective plane (the quotient of the boundary of regular icosahedron by the antipodal involution), a 9-vertex triangulation of the complex projective plane (Kühnel, 1983) and 15-vertex triangulations of the quaternionic projective plane (Brehm and Kühnel, 1992). Recently the speaker has constructed first examples of 27-vertex triangulations of manifolds like the octonionic projective plane and a lot of new 15-vertex triangulations of the quaternionic projective plane. I will speak about these results and also about symmetry groups of these traingulations.
Bio: Alexander Gaifullin is the Correspondent Member of the Russian Academy of Sciences, a Principal Researcher of Steklov Mathematical Institute of RAS, a Professor of Lomonosov Moscow State University (Faculty of Mechanics and Mathematics), and a Professor of Skolkovo Institute of Science and Technology. He was an invited speaker at the European Congress of Mathematics in 2012 and a plenary speaker at the European Congress of Mathematics in 2016. Alexander Gaifullin graduated from Moscow State University in 2005, defended his PhD thesis in 2008 (supervisor: Prof. Buchstaber), and doctoral thesis in 2010.
******
Lecture Series 54 —— December 7, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/DB945BAE16350718DCAF1DB28506BA3C
Valid Until: 2024-01-06 23:59
Lecture 1 —— Axial algebras of Jordan type
Speaker: Ilya Gorshkov (Sobolev Institute of Mathematics)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Axial algebras are a class of non-associative commutative algebras whose properties are defined in terms of the fusion law. When this fusion law is graded, the algebra has a naturally associated automorphism group, and thus axial algebras are related to group theory. Examples of axial algebras include most Jordan algebras and the Grice algebra. In this talk, we introduce the notion of axial algebra and concentrate on axial algebras of Jordan type.
Bio: Professor Ilya Gorshkov is a world-renowned specialist in the field of the theory of finite groups and non-associative algebra. In 2019, he completed the proof of the validity of the famous Thompson conjecture about the set of sizes of conjugacy classes in finite groups. He obtained his PhD degree in 2013 and his Doctor of Science degree in 2020, both from the Sobolev Institute of Mathematics. From 2014 to 2016, he held a postdoctoral position at the University of São Paulo. He currently works at the Sobolev Institute of Mathematics.
Lecture 2 —— Cluster Algebras and Higher Structures
Speaker: Fan Qin(Shanghai Jiao Tong University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We will use topological models to introduce cluster algebras, which are algebras with combinatorial structures. One fundamental problem in studying these algebras is looking for good bases. We will present how their generic bases (dual semi-canonical bases) arise from Calabi-Yau categories and how their triangular bases (Kazhdan-Lusztig type bases) arise from monoidal categories.
Bio: Fan Qin is an associate professor at Shanghai Jiao Tong University, China. He received his PhD from University Paris Diderot (Paris 7), France. His research focuses on algebra and representation theory, with particular emphasis on cluster algebras, their categorifications, and their connections to other fields such as Lie theory, combinatorics, geometry, and topology.
******
Lecture Series 53 —— November 9, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/#/link/251ECA2F394CD626F9F0D59603264344
Valid Until: 2023-12-14 23:59
Lecture 1 —— Random Horseshoe of Anosov systems driven by a quasi-periodic forcing
Speaker: Lian Zeng(Sichuan University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Consider C2 Anosov systems on a compact manifold driven by a quasiperiodic forcing. We study their dynamical complexity on various levels from both perspectives of path-wise dynamics and stochastic processes. In this talk, I will report the results on the existence of random horseshoe from two different viewpoints:topology and probability. This is joint with Wen Huang and Kening Lu.
Bio: Dr. Lian Zeng is currently a professor at the College of Mathematics, Sichuan University. He is mainly engaged in research in dynamic systems and ergodic theory, including smooth ergodic theory of random or infinite dimensional dynamic systems, chaos theory, and ergodic optimization theory. Related work has been published in journals such as MAMS, JAMS, JDE, Adv. Math., Sci. China Math. etc.. He was awarded the 2017 China National Science Funds for Distinguished Young Scientists.
Lecture 2 —— A fresh look at the shadowing of pseudo-trajectories
Speaker: Michael Blank(IITP RAS + HSE)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The problem of shadowing of pseudo-trajectories was inspired by the classical results of D.V.Anosov on the robustness of uniformly hyperbolic systems. The main difficulty here lies in the analysis of infinitely many (in time) perturbations of the system. I will discuss a new approach to the shadowing problem that allows us to overcome this difficulty and expands dramatically its applicability to irreversibleand discontinuous systems, as well as to more general types of perturbations (for example, Gaussian).
Bio: Prof. Michael Blank is one of the most known Russian experts in Probability Theory, Dynamical Systems, Mathematical Physics and Ergodic Theory, and author of a series of breakthrough results in this areas. He has got his PhD degree at 1986 and D.S. degree at 1998. Starting from 2013, Prof. Blank works at Kharkevich Institute for Information Transmition Problem (one of the most famous research centers in Russia where 3 Fields Prize winners worked at). Prof. Blank combines heading a laboratory at this institute and being a Professor of Higher School of Economics.
******
Lecture Series 52 —— October 26, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/38AA9D9EF3F2BA33409D2C4504ABE900
Valid Until: 2023-11-29 23:59
Lecture 1 —— Two dimensional percolation and Liouville quantum gravity
Speaker: Xin Sun(Peking University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Smirnov's proof of Cardy's formula for percolation on the triangular lattice leads to a discrete approximation of conformal maps, which we call the Cardy-Smirnov embedding. Under this embedding, Holden and I proved that the uniform triangulation converge to a continuum random geometry called pure Liouville quantum gravity. There is a variant of the Gaussian free field governing the random geometry, which is an important example of conformal field theory called Liouville CFT. A key motivation for understanding Liouville quantum gravity rigorously is its application to the evaluation of scaling exponents and dimensions for 2D critical systems such as percolation. Recently, with Nolin, Qian and Zhuang, we used this idea and the integrable structure of Liouville CFT to derive a scaling exponent for planar percolation called the backbone exponent, which was unknown for several decades.
Bio: Xin Sun got the bachlor degree in mathematics in 2011 from Peking University, the Ph.D degree in mathematics from MIT in 2017. He spent three years in Columbia University as a junior fellow in the Simons Society of Fellows. Since 2020, he is an assistant professor at University of Pennsylvania. Starting from 2023 fall, he is an tenured associated professor at Beijing International Center for Research in Mathematics at Peking University. His research field is probability and mathematical physics, mainly focusing on random geometry, statistical physics, and quantum field theory.
Lecture 2 —— Nonequilibrium flow modelling: from weak perturbations towards extremal deviations from equilibrium
Speaker: Elena Kustova(Saint Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Nonequilibrium gas states arise in many problems of high-altitude aerodynamics, gas dynamics, low-temperature plasma, and micro-mechanical systems. The degree of deviation from equilibrium is determined by the gas rarefaction, as well as the relationship between the characteristic times of physical and chemical processes occurring in the gas and the time of change in the macroscopic flow characteristics. Depending on the kinetic scaling, models of various complexity can be developed.
This talk is devoted to the construction and implementation of mathematical models of nonequilibrium flows using a continuum approach based on the generalized Chapman-Enskog method, taking into account fast and slow processes. The following topics will be discussed: 1) Models of weakly nonequilibrium flows taking into account relaxation processes at the level of transport coefficients, in particular, bulk viscosity; 2) Models of highly nonequilibrium flows (state-to-state and multi-temperature) and the ways of improving their numerical efficiency; 3) Slip boundary conditions taking into account complex state-to-state vibrational-chemical kinetics in the gas phase and recombination/deactivation reactions on the surface.
Bio: Elena Vladimirovna Kustova is the Acting Head of the Faculty of Mathematics and Mechanics at Saint Petersburg State University (from 2022) being also head of the Department of Fluid Mechanics and Aerodynamics. She graduated from Leningrad University in 1988 and got her PhD degree in 1993, DS degree in Mathematics in 2002. Starting from 2002 he has worked as a professor of the Department of Fluid Mechanics and Aerodynamics and heads this department starting from 2014. Elena Vladimirovna has a honorable degree of Professor of Russian Academy of Sciences. She has a big experience of working as a Visiting Professor in various universities of France, Italy and Brazil.
Prof. Kustova is author of more than 250 publications including two monographs; she mentored more than 50 diploma works and 6 PhD thesis.
Her main research interests are:
- Kinetic theory of transfer and relaxation processes in nonequilibrium reactive gases.
- Construction of strict self-consistent mathematical models of nonequilibrium currents with consideration of complex physico-chemical transformations.
- Research of heat and mass transfer on the surface of aircraft entering the atmosphere of Earth and Mars.
- Study of the speed of non-equilibrium processes.
- Development of software tools for the implementation of models of unequal gas dynamics.
- Methods of machine learning in non-equilibrium aeromechanics.
******
Lecture Series 51 —— October 12, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/EF81527EE21CFF2099C132AC698BE75A
Valid Until: 2023-11-22 23:59
Lecture 1 —— RC-positivity and the geometry of compact complex manifolds
Speaker: Xiaokui Yang(Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, we will present some recent progress on the geometry and topology of compact complex manifolds with RC-positive tangent bundles.
Bio: Xiaokui Yang is currently a Professor at Yau Mathematical Sciences Center and department of mathematics, Tsinghua University. In 2012, he received his Ph.D. from University of California, Los Angeles. After graduation, Xiaokui Yang worked at Northwestern University as an Boas assistant professor. Before joining Tsinghua University, he was a professor at Institute of Mathematics, Chinese Academy of Sciences. His research interests lie in complex geometry, geometric analysis and complex algebraic geometry.
Lecture 2 —— Geometry of Gromov-Hausdorff Distance: State of the Art
Speaker: Alexey Tuzhilin(Moscow State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We discuss classical and modern results devoted to investigation of geometry of the proper class consisting of all metric spaces and endowed with Gromov-Hausdorff distance.
Bio: Alexey Tuzhilin graduated from the Faculty of Mechanics and Mathematics of Moscow State University and defended his doctoral dissertation in 1997. From 1989 to 1992 he worked in the Laboratory of Renewable Energy Sources of the Faculty of Geography. In 1992, he became an assistant professor at the newly created department of Differential Geometry and Applications (Faculty of Mechanics and Mathematics) under the leadership of Anatoly Fomenko, and since 2000 he has been a full professor. In 2001, for a series of works on the theory of ramified extremals of one-dimensional variational problems (together with Alexander Ivanov), he was awarded the Shuvalov Prize of the first degree. He was a member of the expert council of the Higher Attestation Commission, as well as a member of the academic council at the Faculty of Mechanics and Mathematics of Moscow State University. Currently heads the Laboratory of Computer Methods in Natural Sciences and Humanities. He also serves on the editorial boards of several international journals.
******
Lecture Series 50 —— June 22, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/88528D27D91D084510E18D2A213A35EF
Valid Until: 2027-07-31 23:59
Lecture 1——Full horseshoe for the Galerkin truncations of 2D Navier-Stokes equations with degenerate stochastic forcing
Speaker: Wen Huang (University of Science and Technology of China)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, we will introduce the existence of full horseshoe for the Galerkin truncations of 2D Navier-Stokes equations with degenerate stochastic forcing (Hypoelliptic condition). We will also review weak horseshoe and semi-horseshoe. This is based on joint work with Jianhua Zhang.
Bio: Wen Huang is a professor of Mathematics at the University of Science and Technology of China (USTC). In 2003, he received his Ph.D. from the Department of Mathematics, USTC.
Prof. Huang is engaged in the research of the complexity theory of dynamical systems and its application in combinatorial number theory and differential equations. In recent years, he and collaborators have made progress in entropy and Sarnak's Möbius disjointness Conjecture, Multiple Recurrence and Multiple Ergodic theory, and the Fokker-Planck equation and its stationary measure: (1) it is proved that under both deterministic and random frameworks, positive entropy systems have weak horseshoes, partially hyperbolic systems with positive entropy have semi-horseshoes and subpolynomial average complexity systems satisfy Sarnak's Möbius disjointness Conjecture; (2) Prove the pointwise Multiple Ergodic Theorem for ergodic distal systems. The characteristic factor theory of minimal systems was established. (3) A new metric is constructed in the space of all probability measures on a finite graph, from which the Fokker-Planck equation is established. Related work has been published in journals such as CPAM, JEMS, MAMS, Adv. Math, CMP, and so on.
He was awarded the 2012 China National Science Funds for Distinguished Young Scientists; the 2018 National Ten Thousand Talent Program Leading Scientists, P.R.China; the 2018 Second Class National Natural Science Award, P.R.China (ranked 2); the 2021 18th Chen Shing-Shen Mathematics Prize (issued by the Chinese Mathematical Society).
Lecture 2——Review of the behaviour of a model of type many predators - one prey
Speaker: G. J. Söderbacka (Åbo Akademi)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract:


Bio: Prof. Gunnar Söderbacka is a brilliant expert in differential equations and, especially in their applications including mathematical biology, population dynamics, epidemics modelling etc. having a wide range of publications in all these areas. He is closely related to Saint Petersburg mathematical school. He obtained PhD in Leningrad University under the supervision of Acad. V.A. Pliss (Prof. Söderbacka has also got a PhD from Åbo Akademi). He was working in Finnmark University College in Norway, Luleå University of Technology in Sweden, and the Academy of Sciences of Finland.
******
Lecture Series 49 —— June 8, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/AA3FBCE528DCEDB51ACB6A1C97BCB41A
Valid Until: 2027-07-31 23:59
Lecture 1——Bershadsky-Cecotti-Ooguri-Vafa invariants and birational geometry of Calabi-Yau manifolds.
Speaker: Lie Fu (University of Strasbourg)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Bershadsky-Cecotti-Ooguri-Vafa (BCOV) invariants are certain analytically defined invariants for Calabi-Yau manifolds. In this talk, I will report on my joint work with Yeping Zhang proving their birational invariance. This is an analogue of the birational invariance of Betti and Hodge numbers of Calabi-Yau manifolds, a celebrated result due to Batyrev and Kontsevich. Time permitting, I will briefly explain the extension of the theory for mildly singular Calabi-Yau varieties, and discuss some open questions.
Bio: Lie Fu is currently a research fellow at the University of Strasbourg Institute for Advanced Study (USIAS). He obtained PhD in mathematics in 2013 at Sorbonne University under the supervision of Professor Claire Voisin. After spending one semester as member of the Institute for Advanced Study (Princeton) in 2014, he returned to France to take up the position of associate professor at the Claude Bernard Lyon 1 University (2014-2019). From 2019-2021, he was a Radboud Excellence Fellow and remained at Radboud University (Netherlands) as an assistant professor until 2022. The research field of Lie Fu is algebraic geometry. Central objects of his research are Calabi-Yau manifolds and hyper-Kähler manifolds.
Lecture 2——Right-angled polytopes, hyperbolic manifolds and torus actions
Speaker: Taras Panov (Lomonosov Moscow State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A combinatorial 3-dimensional polytope P can be realised in Lobachevsky 3-space with right dihedral angles if and only if it is simple, flag and does not have 4-belts (4-prismatic circuits of facets). This criterion was proved in the works of A.Pogorelov and E.Andreev of the 1960s. We refer to combinatorial 3-polytopes admitting a right-angled realisation in Lobachevsky 3-space as Pogorelov polytopes. The Pogorelov class contains all fullerenes, i.e. simple 3-polytopes with only 5-gonal and 6-gonal facets.
There are two families of smooth manifolds associated with Pogorelov polytopes. The first family consists of 3-dimensional small covers (in the sense of M.Davis and T.Januszkiewicz) of Pogorelov polytopes P, also known as hyperbolic 3-manifolds of Loebell type. These are aspherical 3-manifolds whose fundamental groups are certain extensions of abelian 2-groups by hyperbolic right-angled reflection groups in the facets of P. The second family consists of 6-dimensional quasitoric manifolds over Pogorelov polytopes. These are simply connected 6-manifolds with a 3-dimensional torus action and orbit space P. Our main result is that both families are cohomologically rigid, i.e. two manifolds M and M' from either family are diffeomorphic if and only if their cohomology rings are isomorphic. We also prove that a cohomology ring isomorphism implies an equivalence of characteristic pairs; in particular, the corresponding polytopes P and P' are combinatorially equivalent. This leads to a positive solution of a problem of A.Vesnin (1991) on hyperbolic Loebell manifolds, and implies their full classification.
Our results are intertwined with classical subjects of geometry and topology such as combinatorics of 3-polytopes, the Four Colour Theorem, aspherical manifolds, a diffeomorphism classification of 6-manifolds and invariance of Pontryagin classes. The proofs use techniques of toric topology.
This is a joint work with V. Buchstaber, N. Erokhovets, M. Masuda and S. Park.
Bio: Taras Panov is a Professor at Higher School of Economics in Moscow and Moscow State University (Faculty of Mechanics and Mathematics). He is a renowned expert in algebraic geometry, algebraic topology, and combinatorial geometry author of three monographs and a big number of papers in these areas.
Prof. Panov graduated from Moscow State University in 1996, defended his PhD thesis in 1999 (supervisor: Prof. Buchstaber), and doctoral thesis in 2009. Before getting his Professor position at MSU, Taras Panov has got postdoc positions at Osaka City University and University of Manchester.
Besides, Prof. Panov is laureat of various prestigeous prizes and awards on national and international levels.
******
Lecture Series 48 —— May 25, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/C0611FF18351179DC19875AD9B489896
Valid Until: 2027-06-30 23:59
Lecture 1——Stein's Method: A New Perspective on Normal and Non-normal Approximation
Speaker: Qi-Man Shao (Southern University of Science and Technology)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The classical proof of the central limit theorem is based on the characteristic function or the Fourier transform. It works well for sums of independent random variables. The Stein method is a completely novel approach that works not only for independent random variables but also for dependent random variables. It works for both normal and non-normal approximation. It can also provide the accuracy of approximation. In this talk, we will give a brief review on the fundamentals of Stein's method and recent developments in this area.
Bio: Qi-Man Shao, Chair Professor at Southern University of Science and Technology, China. His main research areas are the limit theory in probability and the asymptotic large sample theory in statistics. He has made fundamental contributions to the self-normalized limit theory and the Stein method for normal and non-normal approximation. Noticeable honors and professional services include: an invited speaker at the ICM 2010 in Hyderabad; IMS Medallion Lecturer, a Keynote Speaker at the 2011 Joint Statistical Meetings; State National Science Award (2nd class) (2015); co-Editor, The Annals of Applied Probability (1/2022 - 12/2024); Associate Editor, Bernoulli (1/2013 - 12/2021); Associate Editor, The Annals of Statistics (11/2003 - 12/2012).
Lecture 2——On the properties of weak solutions to elliptic equations with a drift term
Speaker: Tim Shilkin (St. Petersburg Branch of Steklov Mathematical Institute of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We investigate weak solutions to the Dirichlet problem for an elliptic equation with a drift term having a sign-defined divergence. Under minimal assumptions on the smoothness of the drift, we present results on the existence, uniqueness and local properties of weak solutions, as well as the possible relation of these results with the Navier-Stokes theory. Based on a joint work with M. Chernobai.
Bio: Tim Shilkin is a senior researcher at V.A. Steklov Mathematical Institute of RAS, St.-Petersburg, and at present, he is a visiting researcher at Max Planck Institute for Mathematics in the Sciences, Leipzig. After defending his Ph.D. thesis at the Steklov Institute in St. Petersburg, he joined the research group of Prof. O.A. Ladyzhenskaya at the same institute. His research interests lie in the area of PDEs and mathematical hydrodynamics. He is a recognized expert on the Navier-Stokes theory.
******
Lecture Series 47 —— May 11, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/CA626B9C285F1BD1B7E7508A374BA1B0
Valid Until: 2027-06-30 23:59
Lecture 1——On superintegrability.
Speaker: Nicolai Reshetikhin (UC Berkley, Tsinghua University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The notion of superintegrability in Hamiltonian mechanics generalizes Liouville integrability in a natural way. Roughly, superintegrable systems have an extra "hidden symmetry". Historically, the first example of such a system is the Kepler system with an extra symmetry generated by what is known now as Lenz vectors. The first part of the talk will be focused on geometric structures related to superintegrability. The second part aims at the corresponding quantum notion. It turns out that known constructions from Lie theory and representation theory typically produce superintegrable systems.
Bio: Nicolai Reshetikhin is a Professor of Mathematics at the Department of Mathematics of UC Berkley and Yau Mathematical Research Center of Tsinghua University. He graduated from Leningrad University and obtained PhD at Leningrad Branch of Steklov Mathematical Institute. His research interests lie at the interface of mathematical physics, geometry and representation theory, more specifically in quantum field theory, statistical mechanics, geometry and low-dimensional topology, and representation theory of quantum groups. Nicolai Reshetikhin is an invited speaker of ICM 1990 in Kyoto and a plenary speaker of ICM 2010 in Hyderabad.
Lecture 2——Geometric methods to solving Schläfli differential equations
Speaker: A.D. Mednykh (Sobolev Institute of Mathematics of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract:
[1] Д.А. Деревнин, А.Д. Медных, Объем куба Ламберта в сферическом пространстве // Матем. заметки, Т. 86, вып. 2 (2009), 190-201.
[2] N. Abrosimov, A. Mednykh, Volumes of Polytopes in Spaces of Constant Curvature // Fields Inst. Commun., Vol. 70 (2014), 1-26.
[3] N. Abrosimov, A. Mednykh, Geometry of knots and links // IRMA Lectures in Mathematics and Theoretical Physics, Vol. 33, Topology and Geometry, (2021), 433-454.
[4] A.D. Mednykh, Volumes of two-bridge cone-manifolds in spaces of constant curvature // Transform Groups, Т. 26, вып. 2 (2021), 601-629.
Bio: Alexander Mednykh is a Principal Researcher and the Head of Laboratory of Complex Analysis at Sobolev Institute of Mathematics. He is also a Professor and the Head of Chair at Department of Mathematics of Novosibirsk State University. His fields of interest are Complex Analysis, Riemann Surface Theory, Discrete Groups, Geometry of Three-Manifolds, Knot Theory, Graph theory and Combinatorics. He graduated from Novosibirsk State University in 1974 and obtained his Ph.D. and Doctor of Science degree in mathematics at the Sobolev Institute of Mathematics. He has 150 publications and 15 defended Ph.D students.
******
Lecture Series 46 —— April 27, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/C7DCC1DD2C9C640F862DE94FD472BFC5
Valid Until: 2027-05-31 23:59
Lecture 1——On Banach's isometric subspace problem
Speaker: Sergey Ivanov (St. Petersburg Department of Steklov Mathematical Institute)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: An old problem by Banach asks whether a finite-dimensional Banach space V is necessarily Euclidean if all its hyperplanes are isometric to one another. An equivalent formulation is whether a centered convex body is necessarily an ellipsoid if all its central cross-sections are affine equivalent. The problem has been solved in some dimensions but the general case remains open. We will discuss a differential geometric approach to the problem, its connections to Finsler geometry, and a recent solution of the case dim V=4, obtained jointly with D.Mamaev and A.Nordskova.
Bio: Sergei Ivanov is a Principal Research Fellow at St. Petersburg Department of Steklov Math Institute and the Dean of the Mathematics and Computer Science Department of St.Petersburg State University. He graduated from St.Petersburg State University in 1994, got his PhD in 1996 and Habilitation in 2009. His main research interests are in geometry and dynamical systems. He is a corresponding member of the Russian Academy of Sciences since 2011. He was an invited speaker at the ICM 2010 in Hyderabad. In 2014, he and his co-authors D.Burago and Yu.burago received the Leroy P. Steele Prize from the A.M.S. for their book "A Course in Metric Geometry".
Lecture 2——Coherent sheaves, superconnection, and the Riemann-Roch-Grothendieck formula.
Speaker: Shu Shen (IMJ-PRG)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In this talk, I will explain a construction of Chern character for coherent sheaves on a closed complex manifold with values in Bott-Chern cohomology. I will also show a corresponding Riemann-Roch-Grothendieck formula, which holds for general holomorphic maps between closed non-Kahler manifolds. Our proof is based on two fundamental objects: the superconnection and the hypoelliptic deformations. This is a joint work with J.-M. Bismut and Z. Wei arXiv:2102.08129.
Bio: Shu Shen is a lecturer at Sorbonne University and a researcher at IMJ-PRG. He received his MA at Ecole Polytechnique in 2010 and PhD at Paris-Sud University in 2014. He had research and postdoc experiences at Max-Plank (Bonn), Humboldt University and Paris-Sud University. Shen’s main research interests are geometry and analysis of manifolds. https://webusers.imj-prg.fr/~shu.shen/
******
Lecture Series 45 —— April 13, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/D13210BBC42F4A64DA49AA34E4227E7E
Valid Until: 2027-05-31 23:59
Lecture 1——Exponential sums, differential equations and geometric Langlands correspondence
Speaker: Daxin Xu (AMSS CAS)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In 1970s, Dwork established a relationship between the Bessel differential equation and the Kloosterman sums. Such a relationship can be regarded as an instance of the geometric Langlands correspondence for GL_2. In this talk, we will first review some classical results on exponential sums and differential equations, and then discuss some recent progress on generalizations of Dwork's result from the perspective of geometric Langlands correspondence. It is based on joint works with Xinwen Zhu and Kamgarpour-Yi.
Bio: Daxin Xu is an associated professor at the Institute of Mathematics, Academy of Mathematics and Systems Science, CAS. His research interests lie in arithmetic geometry, in particular, p-adic Hodge theory and geometric Langlands program. He received his Bachelor's Degree from Peking University in 2013, and his Ph.D. from Université Paris-Saclay in 2017.
Lecture 2——Morava K(2)-motives and the Rost invariant
Speaker: Viktor Petrov (St. Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A principal homogeneous bundle under the action of a simple algebraic group determines invariants in the Brauer group called Tits algebras. In the case when the group is simply connected these invariants are trivial, but one can define a higher degree invariant called the Rost invariant. It follows from a result by I. Panin that the motives of full flag varieties with coefficients in the Grothendieck group K0 are isomorphic if and only if the Tits algebras generate the same subgroups in the Brauer group. We propose an analog of this result for the case of the Rost invariant; one considers Morava K-theory K(2) instead of K0.
Bio: Viktor Petrov is an associate professor at St. Petersburg State University. He got his PhD degree in 2005 and Dr.Sci. degree in 2022, both from St. Petersburg State University. He was a postdoc at the University of Alberta (Edmonton, AB) and Max Planck Institute (Bonn, Germany). Viktor Petrov was awarded by the St. Petersburg Mathematical Society the prize for young mathematicians and won the "Young Russian Mathematics" contest (twice).
******
Lecture Series 44 —— March 30, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/FE999300C34E3C58E76EAA978AC3313F
Valid Until: 2027-04-30 23:59
Lecture 1——On The Existence of Multi-dimensional Compressible MHD Contact Discontinuities
Speaker: Zhouping Xin (The Chinese University of Hong Kong)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Contact discontinuities for the ideal compressible magnetohydrodynamics (MHD) are the most typical interfacial waves for astrophysical plasmas and prototypical fundamental waves for systems of hyperbolic conservations laws. Such waves are characteristic discontinuities for which there is no flow across the discontinuity surface while the magnetic field crosses transversally, which lead to a two-phase free boundary problem where the pressure, velocity and magnetic field are continuous across the interface whereas the entropy and density may have discontinuities. Some of the major difficulties for the existence of the Multi-dimensional ideal MHD contact discontinuities are the possible nonlinear Rayleigh-Taylor instability and loss of derivatives due to the non-ellipticity of the associated linearized problem. In this talk, I will present the recent work where we have proved the local existence and uniqueness of MHD contact discontinuities in both 2D and 3D in Sobolev spaces without any additional constraints such as Rayleigh-Taylor sign condition or with surface tensions. The key ingredients of our analysis are the Cauchy formula for MHD, the transversality of the magnetic field, and an elaborate viscous approximation. This talk is based on a joint work with Professor Yanjin Wang of Xiamen University.
Bio: Professor Zhouping Xin is an expert in the areas of partial differential equations, mathematical physics, fluid mechanics, nonlinear waves, numerical analysis and numerical methods for PDEs and applied mathematics. He has made some substantial contributions to the stability theory of linear and nonlinear waves, boundary layer theory, multi-dimensional shock wave theory, transonic flows, interfacial wave motions and free boundary problems in fluid dynamics, vacuum dynamics, vortex methods and relaxation methods, transonic flows, and multi-dimensional compressible and incompressible Navier-Stokes systems with more than one hundred eighty publications in leading international research journals. After getting his Ph. D in mathematics from the University of Michigan (Ann Arbor) in 1988, Professor Xin became a Courant instructor at The Courant Institute of New York University, where he was promoted to be a full professor of mathematics in 1995. In 2000, Professor Xin moved from the New York University to the Chinese University of Hong Kong where he has been the William M. W. Mong Professor of Mathematics and the executive director of the Institute of Mathematical Sciences. Professor Xin got many honors including Sloan Research Fellow (1991-1993, USA), Presidential Fellow (1993, NYU, USA); ICM invited speaker (2002); and Morningside Gold Medalist in Mathematics (2004). Professor Xin is the co-editor-in-chief of MAA, associated editor of JMP and M2AS, and a member of the editorial boards for many journals such as M3AS, Kyoto J. Math, Sciences China Mathematics, JHDE, etc.. http://www.ims.cuhk.edu.hk/people/staff/zpxin/
Lecture 2——Systems of Fractional Partial Differential Equations
Speaker: Murat O. Mamchuev (Institute of Applied Mathematics and Automation, KBSC RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In recent years, considerable interest in differential equations of fractional order has been caused by their numerous applications in many fields of science and technology. This circumstance served as an impetus for the development of the theory of boundary value problems for equations and systems of partial differential equations of fractional order, which has now become one of the intensively developing areas of modern mathematics.
We describe classes of well-posed initial and initial-boundary value problems for linear systems of partial differential equations of fractional order not exceeding one. We will show that these systems can be divided into two different types, which differ significantly in terms of setting correct boundary value problems. The first type includes systems with sign-definite eigenvalues of matrix coefficients in the main part. The second type includes systems of matrix coefficients in the main part of which have eigenvalues of different signs.
Bio: Murat O. Mamchuev graduated from Karachay-Cherkess State University in 1998. In 2005, he received the Candidate of Science degree at Kazan State University named after V.I. Ulyanov-Lenin (currently Kazan Federal University). And in 2021 he received the Doctor of Sciences degree at Lomonosov Moscow State University. He is now Head of the Department of Fractional Calculus at the Institute of Applied Mathematics and Automation of Kabardino-Balkarian Scientific Center of the Russian Academy of Sciences. Since 2022, he is a Professor at the Department of Computer Technologies and Information Security of Kabardino-Balkarian State University. Author of more than 100 scientific papers. His research interests include fractional calculus, fractional order differential equations and systems of such equations, methods for analyzing the correctness of statements and solvability of initial and boundary value problems, and mathematical models based on fractional order differential equations. In 2008-2009, M. Mamchuev became the Laureate of the grant in the field of natural and human sciences "Candidates of Sciences of Russian Academy of Sciences" of the Foundation for the Promotion of National Science. Since 2014, he is an Expert in the scientific and technical sphere of the Ministry of Education and Science of the Russian Federation.
******
Lecture Series 43 —— March 16, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/3CD00DB7B77A6C23A67553D7E98F71B1
Valid Until: 2027-04-30 23:59
Lecture 1——Second order fractional mean-field SDEs with singular kernels and measure initial data
Speaker: Xicheng Zhang (Beijing Institute of Technology)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this work, we establish the local and global well-posedness of weak and strong solutions to second-order fractional mean-field SDEs with singular/distribution interaction kernels and measure initial value, where the kernel can be Newton or Coulomb potential, Riesz potential, Biot-Savart law, etc. Moreover, we also show the stability, smoothness and short-time singularity and large-time decay estimates of the distribution density. Our results reveal a phenomenon that for nonlinear mean-field equations, the regularity of the initial distribution could balance the singularity of the kernel. The precise relationship between the singularity of kernels and the regularity of initial values are calculated, which belongs to the subcritical regime in the scaling sense. In particular, our results provide a microscopic probabilistic explanation and establish a unified treatment for many physical models such as the fractional Vlasov-Poisson-Fokker-Planck system, the vorticity formulation of 2D-fractal Navier-Stokes equations, surface quasi-geostrophic models, fractional porous medium equation with viscosity, etc.
Bio: Xicheng Zhang is a professor at the School of Mathematics and Statistics at the Beijing Institute of Technology. He specializes in Stochastic Analysis, in particular, stochastic differential equations. He obtained his PhD in 2000 from Huazhong University of Science and Technology.
Lecture 2——The theory of hidden oscillations and stability of dynamical systems
Speaker: Nikolay Kuznetsov (St. Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The development of the theory of global stability, the theory of bifurcations, the theory of chaos, and new computing technologies made it possible to take a fresh look at a number of well-known theoretical and practical problems in the analysis of multidimensional dynamical systems and led to the emergence of the theory of hidden oscillations which represents the genesis of the modern era of Andronov’s theory of oscillations. The theory of hidden oscillations is based on a new classification of attractors as self-excited or hidden. While trivial attractors (equilibrium points) can be easily found analytically or numerically, the search for periodic or chaotic attractors may turn out to be a challenging problem (see, e.g. famous 16th Hilbert problem on the number and disposition of limit periodic oscillations in two-dimensional polynomial systems which is still unsolved). Self-excited attractors can be easily discovered when observing numerically the dynamics with initial data from the vicinity of the equilibria. While hidden attractors have basins of attraction, which are not connected with equilibria, and their search requires the development of special analytical and numerical methods.
For various applications, the transition of the system’s state to a hidden attractor, caused by external disturbances, may result in undesirable behavior and is often the cause of accidents and catastrophes. For various engineering applications the importance of identifying hidden attractors is related to the classical problems of determining the boundaries of global stability in the space of parameters and in the phase space. Outer estimations of the global stability boundary in the space of parameters and the birth of self-excited oscillations in the phase space can be obtained by the linearization around equilibria and the analysis of local bifurcations and are related to various well-known conjectures on global stability by the first approximation (see, e.g. Andronov’s proof of the conjecture on the Watt regulator global stability by the first approximation, Aizerman and Kalman conjectures). Inner estimations of the global stability boundary can be obtained by classical sufficient criteria of global stability. In the gap between outer and inner estimations, there is an exact boundary of global stability which study requires the analysis of nonlocal bifurcations and hidden oscillations.
This lecture is devoted to well-known theoretical and practical problems in which hidden attractors (their absence or presence and disposition) play an important role [1-7].
References
1 N. Kuznetsov, Invited lecture "Hidden attractors in science and technologies", Academy of Finland, 2021 (https://www.youtube.com/watch?v=-CzGtbfi8g0)
2 Kuznetsov N.V., Theory of hidden oscillations and stability of control systems, Journal of Computer and Systems Sciences International, 59(5), 2020, 647-668 (https://doi.org/10.1134/S1064230720050093)
3 Kuznetsov N.V., Lobachev M.Y., Yuldashev M.V., Yuldashev R.V., Kudryashova E.V., Kuznetsova O.A., Rosenwasser E.N., Abramovich S.M., The birth of the global stability theory and the theory of hidden oscillations, Proc. of European Control Conf. (ECC-2020), St. Petersburg, 2020, 769–774 (https://dx.doi.org/10.23919/ECC51009.2020.9143726)
4 Dudkowski D., Jafari S., Kapitaniak T., Kuznetsov N.V., Leonov G.A., Prasad A., Hidden attractors in dynamical systems, Physics Reports, 637, 2016, 1-50 (https://doi.org/10.1016/j.physrep.2016.05.002)
5 N.V. Kuznetsov, T.N. Mokaev, O.A. Kuznetsova, E.V. Kudryashova, The Lorenz system: hidden boundary of practical stability and the Lyapunov dimension, Nonlinear Dynamics, 102(2), 2020, 713-732 (https://doi.org/10.1007/s11071-020-05856-4)
6 N. Kuznetsov, T. Mokaev, V. Ponomarenko, E. Seleznev, N. Stankevich, L. Chua, Hidden attractors in Chua circuit: mathematical theory meets physical experiments, Nonlinear Dynamics, 111, 2023 5859–5887, https://doi.org/10.1007/s11071-022-08078-y
7 Wang X., Kuznetsov N.V., Chen G., Chaotic Systems with Multistability and Hidden Attractors, Springer, 2021 (https://doi.org/10.1007/978-3-030-75821-9)
Bio: Nikolay V. Kuznetsov graduated from St. Petersburg University in 2001. He received the Candidate of Science degree and the Doctor of Science degree in 2004 and 2016, respectively, both from St. Petersburg University. In 2008, he defended his Ph.D. degree at the University of Jyvaskyla (Finland), where now is Visiting Professor. He is currently a Full Professor (tenured) and Head of the Department of Applied Cybernetics, St. Petersburg University. Since 2018, he is Head of the Laboratory of information and control systems at the Institute for Problems in Mechanical Engineering of the Russian Academy of Science. His research interests include nonlinear control systems, stability and oscillations in dynamical systems, theory of hidden oscillations, hidden attractors, and phase-locked loop nonlinear analysis. Prof. Kuznetsov was included in the worldwide list of Highly Cited Researchers (Web of Science) in 2019–2021. The research group chaired by Prof. Kuznetsov has been awarded the status of the Leading Scientific School (Center of Excellence) in the field of mathematics and mechanics, since 2018. In 2020, he was elected a foreign academician of the Finnish Academy of Science and Letters and was named Professor of the Year in the field of mathematics and physics. He was awarded the St. Petersburg University Prize in 2020 and the Afraimovich Award in 2021 for the theory of hidden oscillations and stability of dynamical systems. In 2022, he was elected a member of the Russian Academy of Science.
******
Lecture Series 42 —— March 2, 2023(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/6E8BAFCA37E53DE67E20C4851B514234
Valid Until: 2027-04-30 23:59
Lecture 1——Tropical objects in sandpiles
Speaker: Nikita Kalinin (St. Petersburg State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: A sandpile model on a graph G is a simple cellular automata. A state of a sandpile model is a function from the vertices of G to non-negative integer numbers, representing the number of grains at each vertex of G. Then a relaxation of a sandpile model is defined as a sequence of topplings: if a vertex of valency k has at least k grains, then it gives one grain to each of its neighbors, one repeats topplings while it is possible.
Surprisingly for a certain initial state (“a small perturbation of the maximal stable state”), the final picture represents tropical curves and tropical hypersurfaces. I will explain all the definitions, show pictures and if time permits, we can speak about ideas in the proofs.
Bio: Nikita Kalinin is currently an associate professor at Guangdong Technicon-Israel Institute of Technology (China). He graduated from St. Petersburg State University and obtained his PhD in mathematics at the University of Geneva. He was a senior scientific researcher at St. Petersburg Branch of the Higher School of Economics. Kalinin’s research interests include geometry, complex analysis, sandpiles, knots, number theory, graph theory etc. Nikita Kalinin was a gold medalist at IMO in 2005 and was awarded the Young Russian Mathematics grant and a Russian Science Foundation grant.
Lecture 2——Collapsed spaces with Ricci local bounded covering geometry
Speaker: Xiaochun Rong (Rutgers University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: A complete Riemannian n-manifold M is called $\epsilon$-collapsed, if every unit ball in M has a volume less than \epsilon (while often a bound on `curvature' must be imposed to prevent a rescaling of metric). In 1978, Gromov classified `almost flat manifolds' (or the `maximally collapsed manifolds' with sectional curvature bounded in absolute value by one and small diameter) ; a bounded normal covering space of M is diffeomorphic to the quotient of a simply connected nilpotent Lie group modulo a manifold up to a co-compact lattice. This result has been a corner stone in the collapsing theory of Cheeger-Fukaya-Gromov in 90's that there is a nilpotent structure on any $\epsilon$-collapsed manifold with bounded sectional curvature, and this theory has found important applications in Metric Riemannian geometry.
We will survey some recent development in generalizing the collapsing theory to $\epsilon$-collapsed manifolds of Ricci curvature bounded below and the (incomplete) universal cover of every unit ball in M is not collapsed. The study of these collapsed manifolds is partially fuelled by many constructions of collapsed Calabi-Yau metrics using certain underlying singular nilpotent fibrations.
Bio: Xiaochun Rong is a distinguished professor at Rutgers University. He received his undergraduate and master's degrees from Capital Normal University (1978-1984), and his Ph.D. from the State University of New York at Stony Brook in 1990. After graduation, he was a Ritt assistant professor at Columbia University, and an assistant professor at the University of Chicago. Then he became a tenured associate professor (1996) and professor (2002) at Rutgers. Professor Rong's research fields are Differential Geometry and Metric Riemannian Geometry. He has made several fundamental contributions to the convergence and collapse theory and their applications, the geometry and topology of a positively curved manifold, and an Alexandrov space. He has published over 50 papers in internationally renowned journals such as Adv. Math., Amer. J. Math., Ann. of Math, Duke Math., GAFA., Invent. Math., J. Diff. Geom, etc.
Professor Rong was rewarded the Sloan Research Fellowship in 1996 and was an invited speaker at the ICM 2002 in Beijing. He was elected a fellow of the American Mathematical Society in 2017.
******
Lecture Series 41 —— December 29, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/E4734C2C04AEB80A182C4C5B38481DB9
Valid Until: 2027-01-31 23:59
Lecture 1——The Instability Index Formula. Application to the Sobolev problem on a rotating top filled with a viscous fluid
Speaker: Andrei A. Shkalikov (Moscow State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract:

Bio: Andrei A. Shkalikov is a Professor at the Department of Function Theory and Functional Analysis of Moscow State University. He obtained his PhD in 1976 and became Doctor of Physical and Mathematical Sciences in 1985. Shkalikov’s scientific interests include the theory of general and differential operators, operator models in hydromechanics and elasticity theory, the theory of operator matrices and beams, asymptotic theory for differential equations, inverse problems of spectral theory. In 2001, he was awarded the first degree Lomonosov Prize for joint works with A.G. Kostyuchenko "Operators in spaces with an indefinite metric and their applications". He is the author of more than 120 papers. Andrei Shkalikov is an Honored Professor of Moscow State University and also the Corresponding Member of the Russian Academy of Sciences.
Lecture 2——Nonlinear Partial Differential Equations of Mixed Type: Analysis and Connections
Speaker: Gui-Qiang G. Chen (University of Oxford)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Three of the basic types of linear partial differential equations (PDEs) are elliptic, hyperbolic, and parabolic, following the standard classification for linear PDEs. Linear theories of PDEs of these types have been considerably better developed. On the other hand, many nonlinear PDEs arising in Mathematics and Science naturally are of mixed type. The solution of some longstanding fundamental problems greatly requires a deep understanding of such nonlinear PDEs of mixed type, especially mixed elliptic-hyperbolic type. Important examples include shock reflection/diffraction problems in fluid mechanics (the Euler equations) and isometric embedding problems in differential geometry (the Gauss-Codazzi-Ricci equations), among many others. In this talk, we will present some old and new underlying connections of nonlinear PDEs of mixed type with the longstanding fundamental problems and will then discuss some recent developments in the analysis of these nonlinear PDEs through the examples with emphasis on developing/identifying unified approaches, ideas, and techniques for dealing with the mixed-type problems. Some most recent developments, further perspectives, and open problems in this direction will also be addressed.
Bio: Professor Gui-Qiang G. Chen is a leading expert in Partial Differential Equations, Nonlinear Analysis, Mathematical Physics, and related areas. He received his Ph.D. from the Chinese Academy of Sciences in 1987 and was a postdoctoral research fellow at the Courant Institute of Mathematical Sciences (NYU) in 1987-89. His recent research interests include nonlinear PDEs of mixed type, hyperbolic conservation laws, nonlinear waves, free boundary problems, geometric analysis, stochastic PDEs, measure-theoretical analysis, weak convergence methods, and entropy analysis. He has published more than 200 original research papers and more than 10 research books. Since 2000, he has delivered more than 300 invited lectures/talks around the world. He is a Member of the Academia Europaea, Member of the European Academy of Sciences, Fellow of the American Mathematical Society, Fellow of the Institute of Mathematics and its Applications, Fellow of the Society of Industrial and Applied Mathematics, was an Alexander von Humboldt Foundation Fellow (2003-07) and Alfred P. Sloan Foundation Fellow (1991-97), and received the 2011 SIAG/Analysis of Partial Differential Equations Prize (SIAM 2011), Royal Society Wolfson Research Merit Award (2009), Chinese National Natural Science Prize (1989), and Chinese Academy of Sciences Award in Mathematics (1988). Currently, he is Statutory Professor in the Analysis of Partial Differential Equations at the Mathematical Institute and Professorial Fellow of Keble College, University of Oxford.
More information can be found at his homepage: https://www.maths.ox.ac.uk/people/gui-qiang.chen
******
Lecture Series 40 —— December 15, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/E5C32793459707574B5D5FA1C4E122DE
Valid Until: 2027-01-31 23:59
Lecture 1——Szegö measures and vibration of Krein strings
Speaker: Roman Bessonov (St. Petersburg State University and PDMI RAS)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: ../../docs/20221213121117112562.pdf
Bio: Roman Bessonov is a vice-dean of the Faculty of Mathematics and Computer Sciences at Saint Petersburg State University which he combines with working at the Saint Petersburg Department of Steklov Mathematical Institute. Roman graduated in 2009 and got his PhD degree in 2013. He is an outstanding expert in Mathematical Analysis including Real and Complex Analysis, Operator theory, Spectral theory and inverse problems for canonical Hamiltonian Systems. Roman Bessonov has obtained a series of breakthrough results in these areas and obtained several prestigious awards. In particular, in 2021, he received ‘Young Russian Mathematician’ prize thus being recognized as one of the nationwide top 5 mathematicians under 40 (in all areas).
Lecture 2——Global axisymmetric Euler flow with rotation
Speaker: Yan Guo (Brown University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Rotation (e.g. Coriolis force) plays an important role in the dynamics of large scale fluid dynamics modeled by incompressible Euler equations. Thanks to decay and dispersive effect from a constant rotation, we establish asymptotic stability smooth axisymmetric incompressible Euler flows in the presence of a constant rotation force with a non-trivial swirl. We will review the recent progress on dispersive effects and global stability for nonlinear PDE, particularly in the framework of control of space-time resonance.
Bio: Yan Guo is currently a L. Herbert Ballou University Professor of Applied Mathematics, Brown University. He received his B.S. from Peking University in 1987 and Ph.D in Mathematics from Brown University in 1993. He was a Courant Instructor from 1993-95. He joined the faculty of the Division of Applied Mathematics at Brown University as an Assistant Professor in September 1995 and was promoted to Professor in 2004. Professor Guo is an A. P. Sloan Research Fellow for 1998-2000. his main research areas include PDE study on kinetic and fluid models for describing dilute gas, interfacial and boundary layer flows, plasma as well as stellar dynamics.
https://appliedmath.brown.edu/people/yan-guo
******
Lecture Series 39 —— December 8, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/B5D9769E8EB78FF9E30359F4D01EF759
Valid Until: 2027-01-31 23:59
Lecture 1——Billiards in polygons, flat surfaces, and dynamics in moduli spaces
Speaker: Anton Zorich (University Paris Cité)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We will start with a discussion of billiards, including Sinai billiards and Ehrenfest wind-tree model. The study of billiards would naturally lead us to flat surfaces and to dynamics in the moduli space of Abelian differentials. I will finish by trying to explain, why the recent Magic Wand Theorem of Eskin-Mirzakhani-Mohammadi and Filip is so astonishing.
Bio: Professor Anton Zorich is an outstanding expert in Geometry, Topology and Mathematical Physics (e.g. he studied close surfaces with flat metric, interval exchange transformations, Teichmüller flows etc.). He graduated from the Faculty of Mechanics and Mathematics of Moscow State University in 1984 and got his PhD degree in 1987 under the supervision of Acad. Sergei Novikov. In 2006, Anton Zorich was an invited speaker at the ICM in Madrid (topic: "Geodesics on flat surfaces"). Now he works as a Distinguished Professor of Mathematics at the Institute of Mathematics of Jussieu and University Paris Cité.
Lecture 2——Dynamics of non-ergodic foliations, Poincare sections and best approximations
Speaker: Yitwah Cheung (Tsinghua University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The study of the dynamics of Teichmuller flows has in large part been inspired by the analogy with homogeneous flows, e.g an analog of Ratner's theorems for unipotent flows attained for the SL(2,R)-action on the moduli space of holomorphic differentials in the celebrated work of Eskin-Mirzakhani-Mohammadi. Interestingly, some results about homogeneous flows and the behavior of their trajectories were inspired by investigations of dynamical properties of foliations of surfaces and Teichmuller geodesic rays. In this talk, I will describe some contributions in this direction pertaining to various concepts in Diophantine approximation such as singular vectors and Khintchine-Levy constant.
Bio: Yitwah Cheung received his PhD in Mathematics in 2000 from the University of Illinois at Chicago under the direction of Howard A. Masur. After holding the position of Ralph Boas Assistant Professor at Northwestern University, he joined San Francisco State University in 2005, where he became Associate Professor in 2010 and Full Professor in 2015. He is a recipient of the Clay Mathematician Liftoff Award (2000) and NSF CAREER Award (2010-2016) and has published research articles that have appeared in Annals of Mathematics (2003,2011), Inventiones (2011) and Duke (2016) and Ann. Ecole Normale Superior (2024, to appear) on a variety of topics, including the ergodic theory of rational billiards, Teichmuller dynamics and Diophantine approximation. In 2018, he joined Tsinghua University as a member of the Yau Mathematical Sciences Center and the Mathematics Department.
******
Lecture Series 38 —— December 1, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/73D7769DE421B64D45229413FADDE205
Valid Until: 2026-12-31 23:59
Lecture 1——On rationally integrable planar dual and projective billiards
Speaker: Alexey Glutsyuk (Ecole Normale Supérieure de Lyon)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: ../../docs/20221128151431652674.pdf
Bio: Professor Glutsyuk is an outstanding expert in real and complex dynamical systems: billiards, analytic theory of ordinary differential equations, holomorphic foliations, groups of transformations, and mathematical models for Josephson’s effect in superconductivity. He graduated from Moscow State University in 1993 and got his PhD in 1996 under the supervision of Yu.S. Ilyashenko. Alexey Glutsyuk obtained his habilitation at ENS Lyon in 2008 and his doctoral degree in Moscow in 2012. He has experience working at the Institute for Advanced Study in Princeton, Paul Sabatier University in Toulouse, Max Plank Institute in Bonn, and the Independent University of Moscow before obtaining his current position at ENS Lyon.
Lecture 2——Dirac operator and positive scalar curvature
Speaker: Guoliang Yu (Texas A&M University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In this talk, I will discuss an index theorem for Dirac operators on manifolds with corners and its application to Gromov's dihedral rigidity conjecture on scalar curvature. I will make this talk accessible to non-experts. This is joint work with Jinmin Wang and Zhizhang Xie.
Bio: Guoliang Yu is the Powell Chair in Mathematics and University Distinguished Professor at Texas A&M University. He was an invited speaker at the ICM 2006 in Madrid. He is a Fellow of the American Mathematical Society and also a Simons Fellow in Mathematics. His research interests include large-scale geometry, K-theory, index theory, manifold topology and geometry, and operator algebras.
******
Lecture Series 37 —— November 17, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/758C625543237B2825C947F49E8D0D05
Valid Until: 2026-12-31 23:59
Lecture 1——Brownian chain break and some exit problems
Speaker: Mikhail Lifshits (St. Petersburg State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Interacting Brownian particles are a popular model for various physical systems where a number of particles is subjected to inter-particle forces and ambient noise.
We investigate in depth the behaviour of one such model studied earlier both by physicists and mathematicians: a finite chain of Brownian particles, interacting through a pairwise quadratic potential, with one end of the chain fixed and the other end pulled away at slow speed.
We study the instant when the chain “breaks”, that is, the distance between two neighboring particles becomes larger than a certain limit. There are three different regimes depending on the relation between the speed of pulling and the Brownian noise. We prove weak limit theorems for the break time and the break position for each regime. The main tools used are weak dependence and Piterbarg-Pickands theorem on Gaussian large deviations.
As a byproduct, for a class of Gaussian stationary processes, we obtain a limit theorem for the last exit time over a slowly growing linear boundary.
The work displays a series of joint works with F.Aurzada, F.Betz, and N.Karagodin.
Bio: Mikhail Lifshits is a professor at his alma mater St. Petersburg State University since 2000 and a board member of Saint Petersburg Mathematical Society. He got his Master degree in 1978, his PhD in 1981 from then Leningrad State University and received Doctor of Science degree in 1993. As an invited professor, M.Lifshits used to teach in France (Strasbourg, Lille, Paris), in USA (Georgia Tech), in Sweden (Linkoping); he also gave courses in Germany and Finland and many other places. He works in various areas of Random Processes Theory such as Gaussian processes, quantization, small deviations, exit probabilities, etc. as well as in applied Geostatistics. Prof. Lifshits wrote the monograph "Gaussian Random Functions" and textbooks "Lectures on Gaussian Processes" (Springer, 2014) and "Random Processes by Example" (World Scientific, 2015) which are extremely popular among students and young scientists. He is now Editor-in-Chief of the journal Probability Surveys and Associated Editor of Journal of Theoretical Probability, Theory of Probability and Applications, and some other journals.
Lecture 2——The random series-parallel graph
Speaker: Zhan Shi(AMSS CAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: I am going to make some elementary discussions on the random series-parallel graph of Hambly and Jordan (2004). Joint work with Xinxing Chen, Bernard Derrida and Thomas Duquesne.
Bio: Zhan Shi is Professor at AMSS, Chinese Academy of Sciences. Before joining AMSS, he was Professor at Sorbonne Université Paris VI. He received a PhD from Sorbonne Université Paris VI, under the joint supervision of Professors Marc Yor and Paul Deheuvels. Zhan Shi's research interests lie in probability theory, stochastic analysis and statistical physics.
******
Lecture Series 36 —— November 10, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/8F9C3126AFDD066C5842C7AB0185D10B
Valid Until: 2026-12-31 23:59
Lecture 1——On the structure and statistics of the set of classical knots
Speaker: Andrei Malyutin (Saint Petersburg Department of Steklov Mathematical Institute)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We study the structure and statistical characteristics of the set of classical knots. Particular points of this study are the statistics with respect to Thurston's classification (satellite/torus/hyperbolic) and the growth rates of the number of knots with respect to various complexity measures on the set of knots. New estimates for the growth rates of the number of prime knots with respect to the crossing number and arc index will be presented.
Bio: Andrei Malyutin is the deputy director of the Saint Petersburg Department of Steklov Mathematical Institute, a Professor at Saint Petersburg State University, having also an honorary degree of Professor of the Russian Academy of Sciences. He obtained his PhD in 2001 and got his Doctoral Degree (habilitation) in 2010. Andrey has some mathematical awards including the first prize in the International Mathematical Olympiad in 1992. His research interests include Geometric Topology, Geometric Group Theory, Dynamical Systems, Random Processes, etc. He provided solutions for a wide range of difficult problems, some of them going back to Markov and Poisson. Andrei is a member of the editorial board of several prestigious journals e.g. Algebra I Analiz.
Lecture 2——Taut foliations of 3-manifolds with Heegaard genus two
Speaker: Tao Li (Boston College)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Let M be a closed, orientable, and irreducible 3-manifold with Heegaard genus two. We prove that if the fundamental group of M is left-orderable then M admits a co-orientable taut foliation. This verifies part of the L-space Conjecture.
Bio: Tao Li is a professor at the Department of Mathematics at Boston College. He specializes in low-dimensional geometry and topology. He obtained his PhD in 2000 from Caltech. After a Bing Instructor at the University of Texas at Austin, he went to Oklahoma State University before moving to Boston. He was awarded twice the Simons Fellowship in 2015 and 2022 and was elected as a Fellow of the American Mathematical Society in 2016. He was an invited speaker at the 2014 ICM in Seoul.
******
Lecture Series 35 —— October 20, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/A060BCDC327122B952F2EE65455EB411
Valid Until: 2026-11-30 23:59
Lecture 1——Singular Weyl's law with Ricci curvature bounded below
Speaker: Xianzhe Dai (University of California, Santa Barbara)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The classical Weyl's law describes the asymptotic behavior of eigenvalues of the Laplace Beltrami operator in terms of the geometry of the underlying space. Namely, the growth order is given by (half of) the dimension and the limit by the volume. The study has a long history and is important in mathematics and physics. In a very recent joint work with S. Honda, J. Pan and G. Wei, we find two surprising types of Weyl's laws for some compact Ricci limit spaces. The first type could have power growth of any order (bigger than one). The other one has an order corrected by logarithm as some fractals even though the space is 2-dimensional. Moreover, the limits in both types can be written in terms of the singular sets of null capacities, instead of the regular sets. These are the first examples with such features for Ricci limit spaces. Our results depend crucially on analyzing and developing important properties of the examples constructed by J. Pan and G. Wei (GAFA 2022).
Bio: Xianzhe Dai is a professor at the Department of Mathematics at the University of California, Santa Barbara. He specializes in Differential Geometry and Geometric Analysis, in particular the Atiyah-Singer index theory. He obtained his PhD in 1989 from Stony Brook University. After a C. L. E Moore Instructorship at MIT, he went to the University of Southern California before moving to UCSB. He was a Sloan Research Fellow from 1993 to 1995.
Lecture 2——Formation of singularities of 2D soliton equations represented by $L,A,B$-triples
Speaker: Iskander Taimanov (Sobolev Institute of Mathematics of RAS)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We expose recent results on a certain geometrical mechanism of the formation of singularities of modified Novikov-Veselov and Davey-Stewartson II equations. The singular solutions are constructed by means of surface theory. These systems are represented by L,A,B-triples and we discuss the relation of such a mechanism to the degeneration of the zero level discrete spectra of the corresponding L-operators.
Bio: Iskander Asanovich Taimanov is an Academician of the Russian Academy of Sciences and an invited speaker of ICM’2022. He graduated from the Faculty of Mechanics and Mathematics of Moscow State University in 1983. In 1987, Iskander Taimanov defended his PhD thesis under the supervision of academician S. P. Novikov. In 1994, he defended his doctoral thesis at Steklov Institute of the Russian Academy of Sciences.
Now Prof. Taimanov works as a Principal Researcher at Sobolev Institute of Mathematics of the Siberian Branch of RAS being also the Chair of the Department of Geometry and Topology of Novosibirsk State University. Acad. Taimanov is a member of the board of directors of the Kazakh-British Technical University and participates on editorial boards of several prestigious journals. In September 2017, Acad. Taimanov was elected to the Presidium of the Russian Academy of Sciences.
Iskander Taimanov is an expert in research concerning geometry, calculus of variations, and soliton theory. Topics of his work are Morse–Novikov theory and Willmore surfaces. He is the author of the textbook Lectures on Differential Geometry.
******
Lecture Series 34 —— October 6, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recordinig: https://disk.pku.edu.cn:443/link/A60F3CA06FB856C9E5BA5A362C8714D0
Valid Until: 2026-11-30 23:59
Lecture 1——Aspects of the Bogomolov conjectures
Speaker: Xinyi Yuan (Beijing International Center for Mathematical Research)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The original Bogomolov conjecture (proved by Ullmo) asserts that a projective curve of genus greater than 1 over a number field has only finitely many points of small Neron-Tate heights. We will introduce this conjecture and the recent developments on other versions of it, including the geometric Bogomolov conjecture and the uniform Bogomolov conjecture.
Bio: Xinyi Yuan is currently a chair professor at Peking University. He got his Bachelor’s Degree from Peking University in 2003, and got his PHD from Columbia University in 2008. Before joining Peking University in 2020, he was an associate professor at UC Berkeley. He specializes in number theory and arithmetic geometry.
Lecture 2——On a conjecture due to J.-L. Colliot-Thelene
Speaker: Ivan Panin (St. Petersburg Department of Steklov Mathematical Institute)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Let $R$ be a regular local ring containing a field, $K$ be its fraction field, $a\in R^{\times}$ be a unit, $n\geq 1$ be an integer, $1/2$ is in $R$. Particularly, we prove the following result. Suppose a is a sum of n squares in K. Then a is a sum of n squares in R. This is a partial case of a conjecture due to J.-L. Colliot-Thelene (1979). The conjecture is solved in positive for regular local rings containing a field.
In more details. If R contains rational numbers, then the conjecture is solved by the speaker in his Inventiones paper (2009). If R contains a finite field and the residue field of R is infinite, then the conjecture is solved by the speaker jointly with K.Pimenov in 2010 in their Doc. Math. paper. If R contains a finite field, then the conjecture is solved by S. Scully in 2018 in his Proceedings of the AMS paper. If time permits, very recent progress in the topic will be discussed.
Bio: Ivan Panin is a Chair of Algebra and Number Theory at Steklov Mathematical Institute at Sankt-Petersburg. He is a Corresponding Member of the Russian Academy of Sciences since 2003. He got his PhD in 1984 under the supervision of Andrei Suslin. Ivan Panin got his Habilitation in 1995. He was an invited speaker at ICM-2018 in Rio de Janeiro. He solved the Grothendieck--Serre conjecture on principal G-bundles (for regular local rings containing a field). He invented (jointly with A. Smirnov) a topic of oriented cohomology theories on algebraic varieties, stated and proved a Riemann--Roch type theorem for oriented cohomology theories. Jointly with G. Garkusha, he realised a project due to V.Voevodsky producing a machinery for computing motivic infinite loop spaces.
******
Lecture Series 33 —— September 22, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/2C3167F7FADFEB83417F8A3A80B9527E
Valid Until: 2026-10-31 23:59
Lecture 1——The pro-Chern-Schwarz-MacPherson class in Borel-Moore motivic homology
Speaker: Fangzhou Jin (Tongji University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We show that the zero-dimensional part of the pro-Chern-Schwarz-MacPherson class defined by Aluffi is equal to the pro-characteristic class in limit Borel-Moore motivic homology. A similar construction also produces a quadratic refinement of this class in the limit Borel-Moore Milnor-Witt homology. This is a joint work with Peng Sun and Enlin Yang.
Bio: Fangzhou Jin is an assistant professor at Tongji University. He obtained his PhD at Ecole Normale Supérieure de Lyon in 2016 under the supervision of Frédéric Déglise. His work is related to foundational aspects of motivic homotopy theory, a theory introduced by Morel and Voevodsky which studies cohomology theories on algebraic varieties using geometric as well as categorical tools by importing ideas from algebraic topology.
Lecture 2——Triangulated categories, weight structures, and weight complexes
Speaker: Mikhail Bondarko (St. Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: (Co)homology functors usually yield certain functors into triangulated categories. I will justify this claim and recall some basics on homotopy categories of complexes and other triangulated categories. Next, I define weight structures on triangulated categories; these were independently introduced by B. and D. Pauksztello. Weight structures give certain filtrations of triangulated categories; the definition is a certain cousin of that of t-structures. I will mention some methods of constructing weight structures as well as interesting "topological" and motivic examples. Weight structures give certain weight complex functors that are "usually" exact; they are also conservative up to "objects of infinitely large and infinitely small weights" (that is, weight complexes only kill extensions of objects of these two sorts). In particular, one has an exact conservative functor from geometric Voevodsky motives into complexes of Chow motives, whereas the corresponding weight spectral sequences vastly generalize Deligne’s ones. Weight complexes also enable one to calculate the corresponding pure functors; some of the latter are quite new and interesting.
The talk can be interesting to anyone who had some experience with (co)homology and categories.
Bio: Mikhail Bondarko is an associate professor at the St. Petersburg State University and also a professor of the Russian Academy of Sciences. He obtained his PhD in 2000 and got his Doctor Degree (habilitation) in 2007. He has some prestigious awards; this includes first prize in the Chinese Mathematical Olympiad in 1994. Currently, Bondarko studies triangulated categories (including motivic ones), weight structures, and t-structures on them. He also has several papers on formal groups, finite flat group schemes, and additive Galois modules.
******
Lecture Series 32 —— June 16, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/003A36414D9928A3A199EFE5BE0DF5E0
Valid Until: 2026-07-31 23:59
Lecture 1——Bounded elementary generation of Chevalley groups
Speaker: Prof. Nikolai Vavilov
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We discuss several results on bounded elementary generation and bounded commutator width for Chevalley groups over Dedekind rings of arithmetic type in positive characteristics. In particular, Chevalley groups of rank \ge 2 over polynomial rings F_q[t] and Chevalley groups of rank \ge 1 over Laurent polynomial F_q[t,t^{-1}] rings, where F_q is a finite field of q elements, are boundedly elementarily generated. We sketch several proofs, using reciprocity laws, symbols in algebraic K-theory, and surjective stability for K-functors. As a result, we establish rather plausible explicit bounds, that do not depend on q and are better than the known ones even in the number case. Using these bounds we can also produce sharp bounds of the commutator width of these groups. We also mention several applications (Kac---Moody groups, first order rigidity, etc.) and possible generalisations. This is joint work with Boris Kunyavskii and Eugene Plotkin.
Bio: Professor Nikolai Alexandrovich Vavilov works at Saint-Petersburg State University, Department of Mathematics and Computer Sciences (he was one of the co-founders of the Department) and at Saint Petersburg Department of Steklov Mathematical Institute. He is one of the best Russian algebraists having a big number of brilliant results in Structure theory and representations of algebraic groups, algebraic K-theory, overgroups of semisimple groups and tori. Prof. Vavilov graduated from Leningrad State University (now Saint Petersburg State University) and got his Doctor Degree (habilitation) in 1988. He has got a big number of prestigious awards and prizes and grew up dozens of students.
Besides, Nikolai Alexandrovich intensively cooperates with Chinese colleagues. He is one of those people who launched the current program of Russian - Chinese collaboration.
Lecture 2——Arakelov geometry over adelic curves
Speaker: Prof. Huayi Chen
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Arakelov geometry is a theory of arithmetic geometry which combines algebraic geometry over an algebraic integer ring and complex analytic geometry to study the arithmetic properties of projective varieties over a number field. In a joint work with Atsushi Moriwaki, by using the adelic point of view of Chevalley and Weil, we extend Arakelov geometry to the setting of projective varieties over an arbitrary countable field. In this talk, I will explain the main ideas behind this theory, and the similarity and differences compared to the classical approach.
Bio: Huayi Chen is currently a Professor at Paris Diderot University and the Mathematics Institute of Jussieu–Paris Rive Gauche. He graduated from Peking University in 2000. In 2003, he got his Master's degree at Pierre and Marie Curie University. Then he got his PhD at Laurent Schwartz Mathematics Center, Ecole Polytechnique in 2006. His research interests include algebraic geometry, Arakelov geometry and Diophantine problems.
******
Lecture Series 31 —— June 2, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/F98451EB7316CD31517990FA148AC092
Valid Until: 2026-07-31 23:59
Lecture 1——On a geometric realization of the center of the small quantum group
Speaker: Prof. Peng Shan (Yau Mathematical Sciences Center)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In Lie theory, the center of many important representation categories admits geometrical interpretations as singular cohomology of certain algebraic varieties. We will explain a new realization of this type, relating the center of the small quantum group to the cohomology of certain affine Springer fibres. As an application, we obtain a conjectural formula for the dimension of the center of the small quantum group. This is based on a joint work with R. Bezrukavnikov, P. Boixeda-Alvarez, and E. Vasserot.
Bio: Peng Shan is currently a Professor at Yau Mathematical Sciences Center, Tsinghua University. Her research interests lie in Geometric Representation Theory. In 2011, she received her Ph.D. from Paris Diderot University. After graduation, Peng Shan worked at MIT as C. L. E. Moore instructor. From 2011 to 2017, she was an associate researcher at the French National Center for Scientific Research. Also, she carried out research at the University of Caen-Normandie and Paris-Sud University. She is also the invited speaker at the ICM (2022). Prof. Shan Peng is on the editorial board of "J. Algebra" and has published many papers in top mathematics journals such as J. Amer. Math. Soc., Invent. Math., Duke Math. J. and Adv. Math.
Lecture 2——Chevalley groups over rings of polynomials
Speaker: Dr. Anastasia Stavrova (Saint Petersburg State University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Let D be a Dedekind domain. In 1977, A. Suslin established that for any N>=3, and any n>=1, one has SL_N(D[x_1,...,x_n])=SL_N(D)E_N(D[x_1,...,x_n]), where E_N(D[x_1,...,x_n]) is the elementary subgroup of SL_N(D[x_1,...,x_n]), i.e. the subgroup generated by the unipotent elementary transformation matrices E+tE_ij, where E is the unit matrix, E_ij is the matrix with 1 at the position i,j and 0 everywhere else, and t is an arbitrary element of the polynomial ring D[x_1,...,x_n]. In particular, this implies that SL_N(Z[x_1,...,x_n])=E_N(Z[x_1,...,x_n]), where Z is the ring of integers. We discuss an extension of this result to all split simple linear algebraic groups (also called Chevalley groups) and to regular rings D of higher dimension.
Bio: Dr. Anastasia Stavrova is an expert in algebraic groups, non-associative algebra, and algebraic K-theory. She is a researcher in the Chebyshev Laboratory and Department of Mathematics and Computer Science at Saint Petersburg State University. Dr. Stavrova earned a specialist degree in mathematics at Saint Petersburg State University in 2005 and got her PhD in 2009 supervised by Nikolai Vavilov. She has got a great experience of working overseas (the University of Leiden, University of Padua, Ludwig Maximilian University of Munich, and the University of Duisburg-Essen). Also, she was Jerrold E. Marsden Postdoctoral Fellow at the Fields Institute in Canada. Anastasia Stavrova obtained a great number of brilliant results in Algebra and related areas. She won the Young Mathematician Prize of the Saint Petersburg Mathematical Society in 2009, the Young Russian Mathematics Scholarship in 2016. In 2018, she has got the G. de B. Robinson Award from the Canadian Mathematical Society.
******
Lecture Series 30 —— May 26, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/BC2A84C65A65A46B5303EFAE1C4212AA
Valid Until: 2026-06-30 23:59
Lecture 1——The non-positive curvature geometry of some fundamental groups of complex hyperplane arrangement complements
Speaker: Jingyin Huang (Ohio State University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: A complex hyperplane complement is a topological space obtained by removing a collection of complex codimension one affine hyperplanes from C^n (or a convex cone of C^n). Despite the simple definition, these spaces have highly non-trivial topology. They naturally emerge from the study of real and complex reflection groups, braid groups and configuration spaces, and Artin groups. More recently, the fundamental groups of some of these spaces start to play important roles in geometric group theory, though most of these groups remain rather mysterious. We introduce a geometric way to understand classes of fundamental groups of some of these spaces, by equivariantly “thickening” these groups to metric spaces which satisfy a specific geometric property that is closely related to convexity and non-positive curvature. We also discuss several algorithmic, geometric and topological consequences of such a non-positive curvature condition. This is joint work with D. Osajda.
Bio: Jingyin Huang is an assistant professor and the Alice Louise Ridenour Wood Chair in Mathematics at Ohio State University. He completed his PhD at New York University in 2015. He is an expert in geometric group theory, which studies the deep connections between the algebraic properties of groups and the geometry of spaces they act on. His research focus on certain rigidity properties of discrete groups (quasi-isometric rigidity, measure equivalence and orbit equivalence rigidity), and the geometry of non-positively curved spaces and groups. He has been awarded a 2022 Sloan Research Fellowship.
Lecture 2——Combinatorics of Lipschitz polytope and beyond
Speaker: Prof. Fedor Petrov
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Let $(X, \rho)$ be a finite metric space. Consider the space of real functions on $X$ with zero mean. Equip it with Lipschitz norm $\|f(x)\|=\max |f(x)-f(y)|/\rho(x, y)$ and consider the unit ball in this norm, which is a certain convex polytope. The question on classifying metrics depending on the combinatorics of this polytope have been posed by Vershik in 2015. In a joint work with J. Gordon (2017), we proved that for generic metric space the number of faces of a given dimension is always the same. This fact is intimately related to regular triangulations of the root polytope (convex hull of the roots of root system $A_n$). In this survey-style talk, we discuss both this phenomenon and further relations of the subject, observed recently by several groups of mathematicians.
Bio: Prof. Fedor Petrov is an expert in Combinatorics, Convex Geometry, Functional Analysis and Geometry of Numbers. Being a former student of Prof. A.M. Vershik, now he plays a leading role in his research group. Prof. Petrov graduated from Saint Petersburg State University in 2004, got his PhD in 2007 and habilitation (Doctor of Sciences degree) in 2018. He is a Professor and a director of one of the educational programs at the Department of Mathematics and Computer Sciences of Saint Petersburg State University combining this with a research position at the Saint Petersburg Department of Steklov Mathematical Institute. Not only Prof. Petrov is an author of a big number of impressive results in the aforementioned areas, but also a jury member of several top mathematical contests for high school and university students. Besides, he is a member of the Council of the St. Petersburg Mathematical Society (since 2018).
******
Lecture Series 29 —— May 19, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/872A119E054BC012A4A81977B8AA410E
Valid Until: 2026-06-30 23:59
Lecture 1——A violation of the uncertainty principle: indicator functions with thin spectrum and uniformly bounded partial Fourier integrals
Speaker: Sergey Kislyakov (St.Petersburg Department of Steklov Mathematical Institute)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Given a set $a$ of finite measure on the real line, it is possible to find a set $b$ with the properties mentioned in the title and differing from $a$ as little as we want in the sense of measure. There is an analog of this claim for more or less general LCA groups. The discussion will be prefaced with a short survey of various results about conditions that may or may not be fulfilled simultaneously for a function and its Fourier transform. This is a joint work with P.Perstneva.
Bio: Sergey Vitalievich Kislyakov is one of the best Russian experts in Harmonic Analysis, Functional Analysis, Interpolation theory, Fourier Analysis and many other related fields, having obtained a great deal of outstanding results in these areas. Besides, he is the author of several books that are extremely popular both among students and mature researchers. Prof. Kislyakov is a Doctor of Mathematics, an Academician of the Russian Academy of Sciences (since 2016), and the Chief Editor of the "Algebra and Analysis" journal. He was Director of the Saint Petersburg Department of Steklov Mathematical Institute.
Lecture 2——Critical Point Sets of Solutions in Elliptic Homogenization
Speaker: Fanghua Lin (Courant Institute, NYU)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In this talk, I shall outline a proof for bounds on H^(n-2)–Hausdorff measure of critical point sets of solutions in elliptic homogenization. The method works for solutions of elliptic equations with Lipschitz coefficients also. This is a joint work with Zhongwei Shen.
Bio: Professor Fanghua Lin is a Silver Professor of Mathematics at the Courant Institute of Mathematical Sciences, New York University. He received his Ph.D. in 1985 from the University of Minnesota. His research interests are in nonlinear partial differential equations, geometric measure theory and applied & geometric analysis. Professor Lin has made significant contributions to the theory of liquid crystals, harmonic maps, Ginzburg-Landau equations and the theory of homogenization. He has also made important contributions to the studies of topological defects, vortices, quantitative unique continuation, nodal and critical sets of solutions of partial differential equations.
******
Lecture Series 28 —— May 5, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/BBD59D040E4E91A35EFFC9E42CEF593A
Valid Until: 2026-06-30 23:59
Lecture 1——Analysis on compressible Navier-Stokes equations with strong boundary layer
Speaker: Tong Yang (City University of Hong Kong)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: It is a classical problem in fluid dynamics about the stability and instability of different hydrodynamic patterns in various physical settings, in particular in the high Reynolds number limit of laminar flow with the boundary layer. However, there are very few mathematical results on the compressible fluid despite the extensive studies when the fluid is governed by the incompressible Navier-Stokes equations. In this talk, we will present a new approach to studying the compressible Navier-Stokes equations in the subsonic and high Reynolds number regime where a subtle quasi-compressible and Stokes iteration is developed. As a byproduct, we show the spectral instability of the subsonic boundary layer. This is a joint project with Zhu Zhang.
Bio: Tong Yang is a Chair Professor of Mathematics at the City University of Hong Kong. He was President of the Hong Kong Mathematical Society from 2016 to 2020. He has been working in the fields of partial differential equations and kinetic theory. He served as an Editor-in-Chief of Analysis and Applications from 2013 to 2017 and is one of the founding Editors-in-Chief of Kinetic and Related Models launched in 2008. He also serves on several international journals, including the Editorial Advisory Board of London Mathematical Society: Bulletin and Journal, and SIAM Journal on Mathematical Analysis. He is a member of the European Academy of Sciences, The World Academy of Sciences and the Hong Kong Academy of Sciences. The awards and distinctions that he has received include the 2nd class State Natural Science Award of China in 2012 and the Croucher Senior Research Fellowship in 2011.
Lecture 2——Large bifurcation supports
Speaker: Prof. Yulij Ilyashenko
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: The talk deals with a general problem of the bifurcation theory on the two-sphere. Consider a vector field with arbitrary degeneracies: complex singular points, multiple limit cycles and polycycles. What part of the phase portrait will bifurcate under a perturbation of this field, and what part will remain unchanged? Not only the degenerated parts of the phase portrait mentioned above will bifurcate; but some generic parts will also. A highly nontrivial question arises: WHO BIFURCATES? The answer will be given in the talk based on joint work with Natalya Goncharuk.
Bio: Prof. Yulij Ilyashenko is one of the most renowned Russian scientists working in Differential Equations, Dynamical Systems and Geometry. He obtained a big number of brilliant results in bifurcation theory and dynamics beyond uniform hyperbolicity. In particular, he proved the finiteness of the number of limit cycles for polynomial vector fields, thus giving a partial solution to Hilbert's 16th problem (the previous proof provided by Dulac contained a serious gap).
Yulij Sergeevich Ilyashenko is the Rector of the Independent University of Moscow, vice-president of Moscow Mathematical Society, and Professor at Moscow State University, Cornell University, Higher School of Economics, and Steklov Mathematical Institute. Besides, Prof. Ilyuashenko is a member of the editorial boards of several prestigious journals including Ergodic Theory and Dynamical Systems, Journal of Dynamics and control systems and Main Editor of Moscow Mathematical Journal.
******
Lecture Series 27 —— April 7, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/C745583AE3B73B5E4E69ABDBAE6C8459
Valid Until: 2026-05-31 23:59
Lecture 1——Extending periodic maps on surfaces over the 4-sphere
Speaker: Prof. Shicheng Wang, Peking University
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The topic indicated by the title has been addressed by Montesinos (1982) and Hirose (2002) using Rohlin intersection form, and by Ding-Liu-Wang-Yao (2012) using spin structures.
Based on the work above, we deduce a more computable criterion for extending periodic maps on surfaces over the 4-sphere.
Some applications are given. Results including:
(1) Let $F_g$ be the closed orientable surface of genus $g$ and $w_g$ be a periodic map of maximum order on $F_g$.
Then $w_g$ is extendable over $S^4$ for some smooth embedding $e: F_g\ to S^4$ if and only if $g=4k, 4k+3$.
(2) For infinitely many primes $p$, each periodic map of order $p$ on $F_g$ is extendable over $S^4$ for some smooth embedding $e: F_g\ to S^4$.
This is joint work with Zhongzi Wang.
Bio: Prof. Shicheng Wang is currently a Distinguished Professor at the Department of Mathematics, Peking University. He received a master's degree from Peking University in 1981 and a doctorate degree from UCLA in 1988. He has won the Shiing-Shen Chern Mathematics Award and the second prize of the National Natural Science Awards (China). Prof. Wang was an invited speaker at the ICM’2002 in Beijing. His research focuses on low-dimensional topology, involving geometric group theory, dynamical systems, and algebraic topology.
Lecture 2——A Mozaic from Geometry, Dynamics, PDEs, and maybe more.
Speaker: Prof. Dimitri Yu. Burago, Pennsylvania State University, State College PA
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: I am going to begin with some unsolved problems, which, in my opinion, deserve more attention that they receive. For some problems, there are partial solutions, which I would try to sketch or at least discuss. I plan then to go to problems where the progress is more substantial, as time permits. What I plan is indeed a mozaic. I am not sure how many topics I would be able to touch upon and which ones would be more of interest, my collaborators include D. Chen, S. Ivanov, B. Kleiner, Ya. Kurylev, M. Lassas, J. Lu, A. Novikov, L. Polterovich. Maybe, I would concentrate оn two or three topics, maybe many more short stories, depending on the reaction.
Bio: Dimitri Yurievich Burago is a worldwide renowned expert in Geometry, Topology and Dynamical Systems. He graduated from Saint Petersburg State University and has got his doctorate in 1994 under the supervision of Prof. Anatoly Vershik. Now he is working at Pennsylvania State University where he received the title of Distinguished Professor in Mathematics. Besides, Prof. Burago received many prestigious prizes including the Leroy P. Steele Prize by the American Mathematical Society. Also, he was an invited speaker of the ICM’1998 in Berlin.
******
Lecture Series 26 —— March 24, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/EE1E0EAC2E77FE3CF97E4E2B48DAAEA3
Valid Until:2026-04-30 23:59
Lecture 1——On Lyapunov exponents of quasi-periodic cocycles
Speaker: Jiangong You (Chern Institute of Mathematics, Nankai University)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Lyapunov exponents are dynamical invariants. Quasi-periodic cocycles are a special important class of dynamical systems having strong background in quantum physics. Lyapunov exponents of a quasi-periodic cocycle depends sensitively on the smoothness of the cocycle, arithmetic property of the frequency and profile of the potential. So far the behind mechanism of the sensitivity has not been completely understood. In this talk, I will discuss the continuity, Holder regularity of the Lyapunov exponent as well as the quantitative version of Avila’s global theory. The talk is based on joint works with L. Ge, S. Jitomirskaya, Y. Wang, X. Zhao and Q. Zhou.
Bio: Jiangong You is a professor at Chern Institute of Mathematics of Nankai University. His research interests include Hamiltonian dynamics (especially KAM theory for Hamiltonian ODEs and PDEs), smooth ergodic theory (especially Lyapunov exponents) and mathematical physics (especially spectral theory of quasi-periodic Schrodinger operators).
Lecture 2——Systems of isometries: dynamics and topology
Speaker: Alexandra Skripchenko
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Systems of partial isometries of the interval represent a simple combinatorial object which appears in topology in connection with measured foliations on a surface (orientable or non-orientable), in dynamics as a nice model to study billiards in rational polygons and in geometric group theory as a way to describe actions of free groups on R-trees. We will discuss several classes of systems of isometries (interval exchange transformations, interval exchange transformations with flips, interval translation mappings, band complexes) and compare their basic dynamical properties: minimality, ergodicity, invariant measures etc. The talk is mainly based on the joint works with Artur Avila and Pascal Hubert and with Serge Troubetzkoy.
Bio: Prof. Alexandra Skripchenko is the Dean of Faculty of Mathematics at Higher School of Economics (HSE), Moscow. She is expert in Dynamical Systems, Low-dimensional Topology and Geometric Group Theory. In particular, she has got some outstanding results concerning interval exchange mappings and interval translation mappings. Having graduated from Moscow State University in 2009, Alexandra Skripchenko has got her PhD in 2012 and works at HSE since 2014.
******
Lecture Series 25 —— March 8, 2022(20:00-22:00 Beijing time / 15:00-17:00 St Petersburg time).
Recording: https://disk.pku.edu.cn:443/link/EDA3BDF8D06B8765C1542BE7278C7D3B
Valid Until: 2026-06-30 23:59
Lecture 1——Sharp asymptotics for arm events in critical planar percolation
Lecture Series 24 —— December 16, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/D51CB6937C05AB71E2B6F5627F6ECB52
Valid Until:2026-04-30 23:59

Prof. Zaporozhets is one of strongest researchers in Probability theory and Geometry, having major interests in Geometry of numbers, Stochastic Geometry, Convex Geometry, Poisson - Voronoi tessellation, zeros of random polynomials and random analytic functions.
Being a student of Acad. Il'dar Ibragimov, one of the most renowned experts in Probability and Statistics, Dmitry graduated from Saint Petersburg State University, defended his PhD thesis in 2005 and Doctoral thesis in 2015 (both thesis were devoted to Random Polynoms).
Later on, Prof. Zaporozhets has got the honorary degree of Professor of Russian Academy of Sciences.
******
Lecture Series 23 —— December 02, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/A863DD4C00B98F1807DB384C8909F353
Valid Until:2026-04-30 23:59
Lecture 1——Gabor analysis for rational functions
Speaker: Prof. Yuri Belov
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Let $g$ be a function in $L^2(\mathbb{R})$. By $G_\Lambda$, $\Lambda\subset R^2$ we denote the system of time-frequency shifts of $g$, $G_\Lambda=\{e^{2\pi i \omega x}g(x-t)\}_{(t,\omega)\in\Lambda}$.
A typical model set $\Lambda$ is the rectangular lattice $\Lambda_{\alpha, \beta}:= \alpha\mathbb{Z}\times\beta\mathbb{Z}$ and one of the basic problems of the Gabor analysis is the description of the frame set of $g$ i.e., all pairs $\alpha, \beta$ such that $G_\Lambda_{\alpha,\beta}$ is a frame in $L^2(\mathbb{R})$. It follows from the general theory that $\alpha\beta \leq 1$ is a necessary condition (we assume $\alpha, \beta > 0$, of course). Do all such $\alpha, \beta $ belong to the frame set of $g$? Up to 2011 only few such functions $g$ (up to translation, modulation, dilation and Fourier transform) were known. In 2011 K. Grochenig and J. Stockler extended this class by including the totally positive functions of finite type(uncountable family yet depending on finite number of parameters) and later added the Gaussian finite type totally positive functions. We suggest another approach to the problem and prove that all Herglotz rational functions with imaginary poles also belong to this class. This approach also gives new results for general rational functions. In particular, we are able to confirm Daubechies conjecture for rational functions and irrational densities.
Lecture 2——Planar Sobolev extension domains and quasiconformal mappings.
Speaker: Yi Zhang
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: In 1979, Goldshtein et al. pointed out that a bounded simply connected planar domain is a W^{1,\,2}-extension domain if and only if it is a quasidisk. This result was later generalized by Jone to all dimensions, showing that uniform domains are W^{1,\,p}-extension domain for any 1\le p<\infty. However, there are simply connected W^{1,\,p}-extension domains in the plane which are not uniform when p\neq 2, pointed out by e.g. Maz'ya. In this talk I will present my joint work with Koskela and Rajala on this problem, and show its connection to the Ahlfors' quasiconformal reflection theorem.
Bio: My main background is in complex analysis and geometric function theory. I have also done works on harmonic analysis, functional analysis, nonlinear elliptic partial differential equations (p-Laplacians and infinity Laplacian), free boundary problems, calculus of variations, eigenvalue problems of p-Laplacians and so on. Especially, I am interested in problems in the intersection of analysis and geometry.
B.S., Math & Applied Math, Beihang University, September 1, 2009 – June 30, 2013.
M.S., Mathematics, University of Jyväskylä (Supervisor: Pekka Koskela), August 1, 2013 – July 30, 2014.
Ph.D., Mathematics, University of Jyväskylä (Supervisor: Pekka Koskela), August 1, 2014 – July 30, 2017.
Doctoral Dissertation: Planar Sobolev extension domains. (Defended on May 5th, 2017)
******
Video: https://disk.pku.edu.cn:443/link/5B3295FADF4652F5B3814A5112999F28
Valid Until:2026-04-30 23:59
Lecture 1——Symmetry Results in Two-Dimensional Inequalities for Aharonov–Bohm Magnetic Fields.
Speaker: Prof. Ari Laptev,
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Lecture 2——Microscopic conservation laws for the derivative nonlinear Schrodinger equation
Speaker: Guixiang Xu (Beijing Normal University)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
******
Lecture Series 21 —— November 04, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/6D11936873F0A5E492B553AC48F3E6C6
Valid Until:2026-04-30 23:59
*****
Lecture Series 20 — October 21, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/90A35E4EF58FF4E7D0841D0217747F5D
Valid Until:2026-04-30 23:59
Lecture 1——Multiplication Between (Local) Hardy Spaces and Their Dual Spaces
Speaker: Yang Dachun
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: It is well known that bilinear decompositions of products of (local) Hardy spaces and their dual spaces play an important role in the study on various problems from analysis. In this talk, we present some recent progresses on such bilinear decompositions of products of (local) Hardy spaces and their dual spaces. Some open questions are also mentioned in this talk.
Bio: Yang Dachun, Professor, School of Mathematical Sciences, Beijing Normal University. Focus on the research of the real-variable theory of function spaces and its applications. Rewarded by National Science Foundation for Distinguished Young Scholars of China in 2005.
Lecture 2 —— Elementwise sub-representations of groups, and their automorphic analogue.
Speaker: Dipendra Prasad, Prof.
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Suppose V and W are two finite dimensional representations of a group G such that for each element g of G, the eigenvalues of g (counted with multiplicity) on V are contained in the eigenvalues of g on W. What can we say about V and W? Such a question appears naturally in Automorphic representations which we will briefly discuss, but then focus on the abstract question on group representations.
Bio: Professor Dipendra Prasad works at the Indian Institute of Technology Bombay (Mumbai, India) having the second position at Saint - Petersburg State University where he heads the Laboratory ‘Modern Algebra and Applications’ at the Department of Mathematics and Computer Science. He obtained his bachelor’s degree from the St. Xavier College, Mumbai in 1978 before moving to the Indian Institute of Technology Kanpur which he completed in 1980. From 1980–1985, Prasad worked as a research scholar at the Tata Institute of Fundamental Research, Mumbai (TIFR Mumbai). He then completed his PhD under the supervision of Benedict Gross at Harvard, in 1989.
Prof. Prasad is a worldwide known expert in Number Theory, Group Theory and Representation Theory (mainly, on Automorphic Representations and the Gan-Gross-Prasad conjecture).
He has got a big number of prestigious awards on national and international levels. In 2018, he was an invited speaker at the ICM in Rio de Janeiro.
*****
Lecture Series 19 — October 7, 2021(19:00-21:00 Beijing time or 14:00-16:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/F1CEAE494B34A35B67CBA8BD214CB2FD
Valid Until:2026-04-30 23:59
Lecture 1——Norm-resolvent convergence to zero-range models with internal structure in models with strong inhomogeneities
Speaker: Alexander V. Kiselev
Time: 19:00-20:00 Beijing time (14:00-15:00 St Petersburg time)
Abstract:


Bio: Dr. Alexander Kiselev, an outstanding expert in Mathematical Physics, whose interested include but not are limited to:
- Functional model for non-selfadjoint linear operators in Hilbert spaces
- Functional analysis;
- Stability Theory and Similarity Theory for non-selfadjoint linear operators;
- Theory of perturbations.
Having graduated from Saint Petersburg State University in 1996 (Faculty of Physics), Dr. Kiselev obtained his PhD degree in 2001. After that he was working in a big number of top-level universities including Dublin Institute of Technology, Universidad Nacional Autónoma de México, University College London, University of Bath, and Lomonosov Moscow State University,
The number of Alexander Kiselev’s collaborators and coauthors is very impressive as well as the number and scientific diversity of his research projects and students.
Now Dr. Kiselev is working at the Department of Mathematics and Computer Science of Saint Petersburg State University.
Lecture 2 —— Elementwise sub-representations of groups, and their automorphic analogue.
Speaker: Mingying Zhong
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: By identifying a norm capturing the effect of the forcing governed by the Poisson equation, we give a detailed spectrum analysis on the linearized Vlasov-Poisson-Boltzmann system around a global Maxwellian. It is shown that the electric field governed by the self-consistent Poisson equation plays a key role in the analysis so that the spectrum structure is genuinely different from the well-known one of the Boltzmann equation. Based on this, we give the optimal time decay rates of solutions to the equilibrium.
Bio: Zhong Mingying, Professor, College of Mathematics and Information Science, Guangxi University. Focus on the spectral analysis, Green's function and fluid dynamical limits of the kinetic equations with external force, such as the Vlasov-Poisson(Maxwell)-Boltzmann systems, and so on. Rewarded by National Natural Science Foundation--Outstanding Youth Foundation, 2019.
*****
Lecture Series 18 — June 3, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/15CD646FE11B67512C30EDD28E594410
Valid Until:2026-04-30 23:59
Lecture 1——Nevanlinna factorization in classes of analytic functions smooth up to the boundary.
Speaker: N.A. Shirokov
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The definition of all objects used in this announcement will be given in the talk. Let an analytical function f belong to the Hardy class Hp in the unit disc D. Then f may be represented as the product, f=IOf, where I is the so-called inner function, it means that |I(z)|<1 for z belonging D, and |I(z)|=1 for almost every z on the unit circle, and the so-called outer function Of is defined by values of |f(z)| on the unit circle. One of those two factors may be absent. The cited statement is the classical result, the factors I and Of are in a sense independent for the functions f from Hp. Let us consider a class X which is contained in H1 and consists of functions f continuous in the closed disc D--. Then f=IOf ,the inner function I is in general discontinuous in D-- what implies that the outer function Of is to compensate the points of discontinuity of the inner function I. The talk is devoted to the concrete way of this compensation and to the specific properties of outer functions belonging to the analytical Holder classes and to the classes of functions of variable smoothness. The consequences about the half-smoothness of an analytical function in comparison with the smoothness of its modulus on the boundary will be given too.
Bio: Professor Nikolai A. Shirokov is a worldwide renowned expert in harmonic and complex analysis which includes the theory of approximations, boundary properties of holomorphic functions and the theory of singular integrals. Having graduated from Saint Petersburg State University in 1971, he has got his Ph.D.in 1973, became Doctor of Science (Saint Petersburg department of Steklov Institute) in 1985 and got his Professor degree in 1988. Professor Shirokov is the author of 82 research publications (Scopus) and a supervisor of a big number of students (both graduate and undergraduate). In 2014 he was officially recognized as ‘the best teacher’ of Higher School of Economics which is one of the most prestigious Russian Universities. N.A. Shirokov heads the department of Math.Analysis of Saint Petersburg State University since 2005 and the Department of Applied Mathematics at Higher School of Economics (Saint Petersburg branch) since 2013.
Lecture 2 —— Factoring Quasiconformal & quasisymmetric mappings
Speaker: Jinsong Liu (Institute of Mathematics, Chinese Academy of Sciences)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: It follows from the Measurable Riemann Mapping Theorem that we can always present a 2-dimensional quasi-conformal mapping as a composition of quasi-conformal mappings with smaller dilatation. In this talk we will construct n (≥3)-dimensional quasi-conformal homeomorphism between Euclidean spaces which admit no minimal factorization in linear, inner, or outer dilatation. If time permits, I will discuss the composition of quasi-symmetric mappings between metric spaces.
Bio: Jinsong Liu is a professor of institute of mathematics, Chinese Academy of Sciences. His research field is complex analysis and Teichmuller theory.
*****
Lecture Series XVII — May 20, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/820DF463C85B66E00CB1FB0C1DDFB027
Valid Until:2026-04-30 23:59
Lecture 1——Fractional Calculus for m-accretive Operators
Speaker: Dr. Maxim Kukushkin, Moscow State University for Civil Engineering; Institute for Applied Mathematics and Automatization
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this report we aim to construct an abstract model of a differential operator with a fractional integro-differential operator composition in final terms, where modeling is understood as an interpretation of concrete differential operators in terms of the infinitesimal generator of a corresponding semigroup. We study such operators as a Kipriyanov operator, Riesz potential, difference operator. Along with this, we consider transforms of m-accretive operators as a generalization, introduce a special operator class and provide a description of its spectral properties.
Bio: Maksim Kukushkin is a specialist in boundary problems, fractional derivatives and operator theory being author of a wide range of publications in all these areas. He has got his PhD degree in 2016 and, after the worked at Moscow State University of Civil Engineering, Kabardino-Balkarian Scientific Center and Saint Petersburg State Transport University.
Lecture 2 —— Cohn-Vossen inequality on certain noncompact Kahler manifolds
Speaker: Prof. Gang Liu, East China Normal University
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We generalize the Cohn-Vossen inequality to certain noncompact Kahler manifolds. This is related to a conjecture of Yau.
Bio:Gang Liu graduated from the University of Minnesota. He was a postdoctoral fellow at the University of California, Berkeley, and an assistant professor at Northwestern University. Now he is a professor at the East China Normal University.
*****
Lecture Series XVI — May 13, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/8A04293383593E6A308D71987895879D
Valid Until:2026-04-30 23:59
Lecture 1——Newton’s aerodynamic problem: an overview of recent results and open questions
Speaker: Alexander Plakhov University of Aveiro (Portugal) and Institute for Information Transmission Problems RAS (Moscow)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Newton (1687) posed the problem of finding a convex axisymmetric bodies of the smallest aerodynamic drag. So, we are looking for the optimal curve which is the generatrix of the body. In 1993, a similar problem was formulated in a wider class of convex (not necessarily symmetric) bodies. This task proved to be much more difficult: it is about finding the optimal surface. The talk will provide an overview of recent results and methods used, and besides, we formulate some open questions. А special attention will be paid to the following statement. If all points of an open (in the relative topology) subset of the boundary of some optimal body are regular (that is, C ^ 1-smoothness holds), then this set does not contain any extreme points of the body. This statement is a strengthening of a similar result (Brock, Ferone, Kawohl, 1996), formulated for C ^ 2-smooth subsets.
*****
Lecture Series XV——April 22, 2021(15:00-17:00 Beijing time or 10:00-12:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/29E2301EB5B100B243E8F3503523C0D5
Valid Until:2026-04-30 23:59
Lecture 1—— POINT PROCESSES AND INTERPOLATION
Speaker: Alexander BUFETOV (CNRS Steklov IITP RAS)
Time: 2021-04-22 15:00-16:00 Beijing time or 10:00-11:00 St Petersburg time
Abstract: The Kotelnikov theorem recovers a Paley-Wiener function from its restriction onto an arithmetic progression. A Paley-Wiener function can also be recovered from its restriction onto a realization of the sine-process with one particle removed. If no particles are removed, then the possibility of such interpolation for the sine-process is due to Ghosh, for general determinantal point processes governed by orthogonal projections, to Qiu, Shamov and the speaker. If two particles are removed, then there exists a nonzero Paley-Wiener function vanishing at all the remaining particles.
How explicitly to interpolate a function belonging to Hilbert space that admits a reproducing kernel, given the restriction of our function onto a realization of the determinantal pont process governed by the kernel? In the case of the zero set of the Gaussian analytic function, or, in other words, the determinantal point process governed by the Bergman kernel, in joint work with Qiu, the Patterson-Sullivan construction is used for uniform interpolation in dense subspaces of the Bergman space. The invariance of our point process under Lobachevskian isometries plays a key rôle.
For the sine-process, the Ginibre process, the determinantal point process with the Bessel kernel and the determinantal point process with the Airy kernel, A.A. Borichev, A.V. Klimenko and the speaker proved that if the function decays as a sufficiently high negative power of the distance to the origin, then the answer is given by the Lagrange interpolation formula.
Bio: Alexander Bufetov is an expert in Ergodic Theory, Probability, Dynamical Systems and Statistics.
He graduated from Moscow State University being student of Acad. Ya. Sinai, one of the worldwide greatest experts in Ergodic Theory. Later on, he has got his PhD from Princeton University. In 2011 he became Doctor of Sciences. In 2015 he won the prestigious Sofia Kovalevskaya price and, thereafter, has got a honorary degree of ‘Professor of Russian Academy of Science’. He is an author of an impressive number of outstanding results https://scholar.google.com/citations?user=nAkXSowAAAAJ&hl=ru.
Prof. Bufetov had a wide range of prestigious positions: at Mathematical Institute of Russian Academy of Sciences, Higher School of Economics, Rice Univeristy, Chebyshev Laboratory at Saint Petersburg State University etc. Now he is working at University Aix-Marseille at CNRS Director position.
Lecture 2—— Differential Network Analysis via Lasso Penalized D-Trace Loss
Speaker: Ruibin Xi (Peking University)
Time: 2021-04-22 16:00-17:00 Beijing time or 11:00-12:00 St Petersburg time
Abstract:
Biological networks often change under different environmental and genetic conditions. In this paper, we model the network change as the difference of two precision matrices and propose a novel loss function called the D-trace loss, which allows us to directly estimate the precision matrix difference without attempting to estimate precision matrices. Under a new irrepresentability condition, we show that the D-trace loss function with the lasso penalty can give consistent estimators in high-dimensional settings if the difference network is sparse. A very efficient algorithm is developed based on the alternating direction method of multipliers to minimize the penalized loss function. Simulation studies and a real data analysis show that the proposed method outperforms other methods.
Bio:
Dr. Ruibin Xi is an associate professor at School of Mathematical Sciences, Peking University. He obtained his PhD from Washington University in St. Louis and received post doc training at Harvard Medical School. His main research interests include statistical analysis of big biological data, cancer genomics, network and graphical models, Bayesian analysis and high-dimensional statistics.
*****
Lecture Series XIV——April 8, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/1D59046D6638F95A152BF678ECE03FC3
Valid Until:2026-04-30 23:59
Lecture 1——Transcendence Theory over Function Fields on Quotients of Bounded Symmetric Domains
Speaker: Ngaiming Mok (The University of Hong Kong)
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Finite-volume quotients of bounded symmetric domains Ω, which are naturally quasi-projective varieties, are objects of immense interest to Several Complex Variables, Algebraic Geometry, Arithmetic Geometry and Number Theory, and an important topic revolves around functional transcendence in relation to universal covering maps of such varieties. While a lot has already been achieved in the case of Shimura varieties by means of methods of Model Theory, Hodge Theory and Complex Differential Geometry, techniques for the general case of not necessarily arithmetic quotients Ω/Γ =: XΓ have just begun to be developed. For instance, Ax-type problems for subvarieties of products of arbitrary compact Riemann surfaces of genus ≥ 2 have hitherto been intractable by existing methods. We will explain how uniformization theorems for bi-algebraic varieties can be proven by analytic methods involving the Poincar´e-Lelong equation in the cocompact case (joint work with S.-T. Chan), generalizing in the absence of the emisimplicity theorem of Andr´e-Deligne for monodromy groups (proven for arithmetic lattices). Klingler-Ullmo-Yafaev (2016) resolved the hyperbolic Ax-Lindemann Conjecture for Shimura varieties in the affirmative ascertaining that the Zariski closure of the image π(S) of an algebraic subset S ⊂ Ω under the universal covering map π : Ω → XΓ is totally geodesic. I will explain how the arithmeticity condition can be dropped in the cocompact case by a completely different proof using foliation theory, Chow schemes, partial Cayley transforms and K¨ahler geometry.
Bio: 莫毅明教授为香港大学明德教授与讲座教授,自1999年始兼任数学研究所所长。莫毅明1980年在斯坦福大学获得数学系哲学博士学位,旋即在普林斯顿大学开展其职业生涯,历任美国哥伦比亚大学正教授与法国巴黎大学(奥赛)正教授,1994年回香港任职香港大学数学系讲座教授。1984年莫毅明获美国斯隆研究基金,1985年获美国总统年青研究人员奖,1998年获香港裘槎优秀科研者奖,2007年获国家自然科学奖二等奖, 2009年获美国数学会伯格曼奖(Bergman Prize).莫毅明为美国数学会会士。莫毅明自1992年起担任“数学年鉴(Mathematische Annalen)”编辑委员,并于2002至2014年期间担任 “数学发明(Inventiones Mathematicae)”编辑委员。莫毅明1994年获邀在苏黎世于国际数学大会(ICM)做学术演讲,并获委任为ICM 2010(海得拉巴)的菲尔兹奖选委。2015莫毅明获选中国科学院院士与香港科学院院士。
Lecture 2 ——A New Life of the Old Sieve
Speaker: Yuri Matiyasevich
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Prime numbers are one of the most important objects of study in Number Theory. Greek mathematician Eratosthenes (276--194 B.C.) invented a method (known nowadays under his name) for revealing primes among all natural numbers. A more modern and powerful for tool for studying prime numbers is Riemann's zeta function. In recent years the speaker performed intensive computer calculations in the search for new properties of this function. Unexpectedly, the Sieve of Eratosthenes naturally appeared in one calculation with the non-trivial zeros of the zeta function. This form of the sieve demonstrates a rich fractal structure lacking in the original sieve. Later another calculation revealed a different kind of a sieve which is dual to the classical Sieve of Eratosthenes. So far no theoretical explanation was found for the observed phenomena. Calculation were not an easy computational task. They required calculations of several thousands of initial non-trivial zeros of the zeta function with several thousands decimal digits, and solving systems consisting of several thousands linear equations.
Bio: 马蒂亚舍维奇教授是当代俄罗斯最著名的数学家之一,圣彼得堡数学学会主席。1966年,19岁的他在逻辑学方面取得了一些突破性的成果,应邀在当年莫斯科举行的国际数学家大会上作邀请报告。1970年,尤里-马蒂亚舍维奇解决了希尔伯特第十问题。到目前为止,他在其他经典领域,如四种颜色问题和黎曼问题上取得了广泛的突破性成果。 他获得了许多著名的奖项,包括洪堡奖、苏联科学院的Markov奖等。他曾获得奥弗涅大学克莱蒙费朗分校和皮埃尔和玛丽居里大学的荣誉学位。Matiyasevich教授是AMS、巴伐利亚科学院和许多其他科学团体的成员。Yuri Vladimirivich Matiyasevich自1970年起在圣彼得堡的斯捷科洛夫研究所工作,1995年成为圣彼得堡国立大学教授。 2002年起,他担任圣彼得堡市数学奥林匹克竞赛负责人。2008年,Matiyasevich教授当选为俄罗斯科学院正式院士。Matiyasevich院士培养了一大批优秀的学生,其中有多位教授和院士。
*****
Lecture Series XIII——March 25, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
To Join Zoom Meeting:
Video: https://disk.pku.edu.cn:443/link/4459C670911CEF7FBFC326AC056FDDC5
Valid Until:2026-04-30 23:59
Lecture 1—— Polynomial complexity and Sarnak conjecture
Speaker: Prof. Wen Huang, University of Science and Technology of China(USTC)
Bio: Huang Wen received his Ph.D. from the Department of Mathematics, USTC in 2003. His research field is topological dynamical system and ergodic theory, related to the entropy and chaos theory, multipleergodic Theorem, Sarnak’s conjecture and so on. He and his collaborators proved the following results: 1) positive entropy implies weak horseshoes. 2) pointwise multiple ergodic theorem holds for distal measure-preserving systems. 3) Sarnak’s conjecture holds for dynamical systems with sub-polynomial measure complexity. He achieved many rewards: 2012 China National Science Funds for Distinguished Young Scientists; 2018 National Ten Thousand Talent Program Leading Scientists, P.R.China; 2018 Second Class National Natural Science Award, P.R.China(rank 2).
Lecture 2 ——Dynamical models of some sociological problems.
Speaker: Sergei Yu. Pilyugin, Faculty of Mathematics and Computer Science,St. Petersburg State University.
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Bio: Sergei Yu. Pilyugin is Former vice President of Saint Petersburg Mathematical Society, he is a professor at Faculty of Mathematics and Computer Science, St. Petersburg State University, Russia. His research interests are in dynamical systems (especially theory of attractors and shadowing theory) and in applications (including sociological models).
*****
Lecture Series Ⅻ —— March 11, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
To Join Zoom Meeting:
Video: https://disk.pku.edu.cn:443/link/11FB200B91B098AB7C5E6A2D07EE6445
Valid Until:2026-04-30 23:59
Lecture 1—— The J-equation and the deformed Hermitian-Yang-Mills equation.
Speaker: Prof. Gao Chen, University of Science and Technology of China.
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The deformed Hermitian-Yang-Mills (dHYM) equation is the mirror equation for the special Lagrangian equation. The "small radius limit" of the dHYM equation is the J-equation, which is closely related to the constant scalar curvature K\"ahler (cscK) metrics. In this talk, I will explain my recent result that the solvability of the J-equation is equivalent to a notion of stability. I will also explain my similar result on the supercritical dHYM equation.
Bio: Chen Gao got Bachelor's degree in Univeristy of Science and Techonology of China in 2012 and Ph.D. in Stony Brook University in 2017. Since then he has worked in IAS and University of Wisconsin-Madison. From January of 2021 he became a tenure-track professor in USTC.
Lecture 2 ——Various types of spectra and spectral measures on Cayley graphs of finitely generated groups and their actions.
Speaker: Tatiana Nagnibeda (University of Geneva)
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: We will be interested in the Laplacian on graphs associated with infinite finitely generated groups: Cayley graphs and more generally, Schreier graphs corresponding to some natural group actions. The spectrum of such an operator is a compact subset of the closed interval [0,2], but not much more can be said about it in general. Little is known about the associated spectral measures either. We will discuss various spectral problems arising in this context and stemming from the famous question “Can one hear the shape of a drum?”
*****
Lecture Series Ⅺ—— January 28, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/5E58EEF73495E01118D5216AE2E12DA6
Valid Until:2026-04-30 23:59
Lecture 1—— On local combinatorial formulas for Euler class of spherical fiber bundle.
Speaker: Dr. Nikolai Mnev, Senior Research Fellow of Chebyshev Laboratory at Saint-Petersburg State University and Saint Petersburg Department of Steklov Mathematical Institute
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: We will discuss the classical problem on local combinatorial formulas of characteristic classes on example of Euler class. Suppose we have a PL spherical fiber bundle with a fiber S^n triangulated over the base simplicial complex. The bundle determines n+1 dimensional Euler characteristic class in the base. Local combinatorial formula for the Euler class is a universal combinatorial function of elementary triangulated S^n-bundles over n+1 simplices universally representing Euler cocycle of the bundle in simplicial cohomology of the base. Such functions exist for rational coefficients in cohomology. They can be constructed as "twisting cochains" -- explicit local chain-level formulas for Gysin homomorphism in the Gysin sequence of the bundle. To get an access to local chain combinatorics of spectral sequence of the bundle we may use Guy Hirsh homology model of the bundle as a local system and then applying homology perturbation theory obtain local formulas as certain measure of twisting in combinatorial Hodge structure of the elementary bundle. The answer can be interpreted and evaluated statistically as certain combinatorial counting using Catanzaro-Chernyak-Klein higher Kirchhoff theorems. The formulas are resulting in a combinatorial form of Gauss-Bonne theorem. For example we easily obtain otherwise difficult to access statement: One can triangulate only trivial and Hopf circle bundles over a 2-dimensional sphere if the base sphere is triangulated as the boundary of 3-simplex.
Bio: Dr. Nikiolay Mnev graduated from the faculty of Mathematics and Mechanics of Saint Petersburg State University in 1980. In 1986 he defended his PhD thesis under supervision of Prof. Anatoly Vershik. Since that time he works at Steklov Institute and, besides, he has got a position at Chebyshev Laboratory starting from its foundation in 2011. Additionally, Dr. Mnev curates the so-called Fizmatklub, the organization intended to boost the level of teaching maths in Saint Petersburg Universities by organizing additional lecture courses, delivered by leading specialists.
Dr. Nikolai Mnev’s research interests cover Algebraic Topology, Geometric Topology and Combinatorial Geometry. In 1991, he received Delbert Ray Fulkerson prize for outstanding papers in the area of discrete mathematics.
Lecture 2 —— Recent developments in exact Lagrangian fillings.
Speaker: Honghao Gao, Michigan State University
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Legendrian knots and their exact Lagrangian fillings are important geometric objects to study in low dimensional contact and symplectic topology. It was not known whether there exists a Legendrian knot that admits infinitely many exact Lagrangian fillings. In 2020, this statement was proven affirmatively, and infinitely many Lagrangian fillings have been constructed for all torus links of infinite type (with Casals), a family of positive braid links (Casals-Zaslow), all positive braid links of infinite type (with Shen-Weng), two examples that are not positive braid links (Casals-Ng). In this talk, I will review these results, and explain the construction for the torus (3,6) link appeared in the work with R. Casals.
Bio: Honghao Gao received his PhD degree from Northwestern University in 2017, under the supervision of Eric Zaslow. He was previously a postdoc at Institut Fourier, and is currently a postdoc at Michigan State University. His research interest is in contact and symplectic topology.
*****
Lecture Series Ⅹ—— January 14, 2021(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/0CA255AFF20FD43771B84685ECA5EEC9
Valid Until:2026-04-30 23:59
Speaker:Dr. Petr. Shpilev
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: The lecture is based on a joint talk with Prof. Vyacheslav Melas. Within the framework of the report, a brief overview of the history of the development of the theory of optimal experiment designs will be given, the basic concepts, definitions, and some key results of this theory are considered.The problem of changing the dimension of the initial optimization task and some approaches to the solution of this problem will be considered on the examples of tasks of constructing specific optimal designs studied by the author of the report.
Bio: Dr. Shpilev graduate from the Faculty of Mathematics and Mechanics in 2004 and got his PhD degree in 2007. His research interests include: Optimal Experimental Design Theory, Regression Analysis, Statistics, Mathematical Simulation, Data Analysis, Computer Science, and Approximation Theory.
Lecture 2 —— Exploring Stochastic Methods For Deep Learning and Reinforcement Learning
Speaker: Zaiwen Wen, Beijing International Center for Mathematical Research.
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Stochastic methods are widely used in machine learning. In this talk, we present a structured stochastic quasi-Newton method and a sketchy empirical natural gradient method for deep learning. We also introduce a stochastic quadratic penalty algorithm for reinforcement learning.
Bio: Wen's research interests include large-scale computational optimization and their applications in data sciences. Together with his coauthors, he has developed both deterministic and stochastic semi-smooth Newton algorithms for composite convex program and Newton type algorithms for Riemannian optimization, as well as academic software packages such as SSNSDP, ARNT, Arrabit, LMSVD and LMAFIT, etc. He was awarded the Science and Technology Award for Chinese Youth in 2016, and the Beijing Science and Technology Prize-Outstanding Youth Scholar Zhongguan Village Prize in 2020. He is an associate editor of Journal of the Operations Research Society of China, Journal of Computational Mathematics and a technical editor of Mathematical Programming Computation.
*****
Video: https://disk.pku.edu.cn:443/link/A59A44F4FDF41825DCBF8AE9578C8D2B
Valid Until: 2025-01-01
*****
Lecture Series Ⅷ ——December 3rd, 2020(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/51D4B1FC28EA321B4C4BC25FEF15C28F
Valid Until: 2025-01-01
Lecture 1——Graph-walking automata.
Speaker:Prof. Alexander Okhotin.
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time) .
Abstract: Graph-walking automata are a model studied in theoretical computer science: they traverse an undirected graph by following its edges, and use a memory of constant size to plan their movements. Graph-walking automata can be regarded both as a model of a robot navigating an unknown environment, and as a generic model of computation, with the graph modelling its memory. This paper presents the known results on these automata, ranging from their limitations in traversing graphs, studied already in the 1970s, to the later work on the logical reversibility of their computations, including the most recent lower bounds on their size.
Bio:Alexander Okhotin (Ph.D. 2004, Queen's University) is a professor of theoretical computer science at St. Petersburg State University. His main research subjects are formal grammars, finite automata and their complexity.
Lecture 2 ——Modeling and Verification of Concurrent and Distributed Systems: From Reo to Mediator.
Speaker: Prof. Meng Sun.
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time) .
Abstract: In this talk I will introduce the channel-based coordination language Reo and the component-based modeling language Mediator, and show how to formalize and verify component-based concurrent and distributed system models in them. Reo provides a channel-based model which focuses on complex interactions among system components. Mediator supports a two-step hierarchical modeling approach: Automata, which provide an interface of ports, are the basic behavior units; Systems declare components or connectors through automata, and glue them together. With the help of Reo and Mediator, both components and systems can be modeled separately and precisely.
Bio:Meng Sun received his BSc and PhD degrees in applied mathematics from Peking University, in 1999 and 2005, respectively. He then spent one year as a postdoctoral researcher in National University of Singapore. From 2006 to 2010, he worked as a scientific staff member at CWI, the Netherlands. He has been a faculty member at Peking University since 2010 and became a full professor in 2017. Currently, his research interests mainly lie in software theory and formal methods. His recent work includes coordination models and languages, coalgebra theory, model checking, theorem proving, software testing, cyber-physical systems, service-oriented and cloud computing, modeling and verification of blockchain and smart contracts, big data analysis, theoretical foundations of machine learning, deep learning and their application in formal verification.
*****
Lecture Series Ⅶ ——November 19th, 2020(20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/B06B1459AA07B80DD51E17F8406655C2
Valid Until: 2025-01-01
Lecture 1——Deep CT Imaging by Unrolled Dynamics
Speaker: Bin Dong, Beijing International Center for Mathematical Research, Peking University
Time: 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: In this talk, I will start with a brief review of the dynamics and optimal control perspective on deep learning (including supervised learning, reinforcement learning, and meta-learning), especially the so-called unrolled dynamics approach and its applications in medical imaging. Then, I will present some of our recent studies on how this new approach may help us to advance CT imaging. Specifically, I will focus on our thoughts on how to combine the wisdom from mathematical modeling with ideas from deep learning. Such combination leads to new data-driven image reconstruction models and new data-driven scanning strategies for CT imaging, and with a potential to be generalized to other imaging modalities.
Bio:Bin Dong received his B.S. from Peking University in 2003, M.Sc from the National University of Singapore in 2005, and Ph.D from the University of California Los Angeles (UCLA) in 2009. Then he spent 2 years in the University of California San Diego (UCSD) as a visiting assistant professor. He was a tenure-track assistant professor at the University of Arizona since 2011 and joined Peking University as an associate professor in 2014. His research interest is in mathematical modeling and computations in imaging and data science. A special feature of his research is blending different branches in mathematics which include: bridging wavelet frame theory, variational techniques, and nonlinear PDEs; bridging differential equations and optimal control with deep learning.
Lecture 2 —— Gaussian random fields in machine learning
Speaker: Viacheslav Borovitskiy, Department of Mathematics and Mechanics,Saint Petersburg State University.
Time: 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: I will introduce the Gaussian process regression algorithm, a widely used approach to probabilistic modeling in machine learning, and talk about its applications and challenges associated with its use. In the end, I will mention our own recent research in this topic that was presented on International Conference on Machine Learning and will be presented on this year's conference on Neural Information Processing Systems.
Bio: He has graduated from Saint-Petersburg State University, Faculty of Mathematics and Mechanics. His main interests cover PDEs, Banach spaces, Satistics and Informatics.
*****
Lecture Series VI - November 5th, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video: https://disk.pku.edu.cn:443/link/E68335CAFDAD6A71A943A348EF8FC42A
Valid Until: 2025-01-01
Speaker: Prof. Yanjun Liu, Jiangxi Normal University
Abstract: The question of which prime powers can occur as divisors of irreducible character degrees of finite groups has a long history. One related conjecture is given by Geoffrey Robinson in 1996, who conjectured that the p-part of character degrees in a p-block of a finite group can be bounded in terms of the center of a defect group of the block. I will mention recent progress on Robinson's conjecture for odd primes,and then turn to the question of when a p-block of a finite group G is also a q-block of G. A series related work have been done by Navarro-Willems,Bessenrodt-Mall-Olsson, Navarro-Turull-Wolf and etc. The block separation property is studied by Bessenrodt-Zhang and they proved that the nilpotency, p-nilpotency of a finite group can be characterized by intersections of principal blocks of some (quotient) groups. Thus it is natural to ask if the solvability or p-solvability of a finite group can also be characterized in this way. By introducing the so-called block graph of a finite group this problem was solved affirmatively. Finally, I will talk about a conjecture relating the trivial intersection of principal blocks to the existence of nilpotent Hall subgroups.
Bio: Dr. Yanjun Liu got Ph. D. from Peking University and is now working in Jiangxi Normal University. Recently He got Humboldt Research Fellowship based on his excellent work in some canonical conjecture of representation theory.
Speaker: Prof. Mikhail Gavrilovich, Saint Petersburg Department of Higher School of Economics
Abstract: We consider simplicial sets equipped with a notion of smallness, and observe that this slight “topological” extension of the “algebraic” simplicial language allows a concise reformulation of a number of classical notions in topology, e.g. continuity, limit of a map or a sequence along a filter, various notions of equicontinuity and uniformconvergence of a sequence of functions; completeness and compactness; in algebraic topology, locally trivial bundles as a direct product after base-change and geometric realisation as a space of discontinuous paths. These reformulations are elementary and can perhaps be used in teaching to give motivated examples of elementary concepts in category theory. Surprisingly, this category is not well-studied and thus these observations raise many easy but open problems, which we like to think are in line with goals of tame topology put by Grothendieck. In the talk, we will work through of a couple of example, briefly mention some others, and indicate a number of open problems, who we like to think are in line with the goals of tame topology put by Grothendieck.
We will preceed the main part of the talk by explaning a category-theoretic characterisaiton of finite solvable and nilpotent groups in terms of the Quillen lifting property, which is also used in reformulations of the notions of limit, compactness, and completeness, and others.
http://mishap.sdf.org/by:gavrilovich/treplo-groups.pdf.
*****
Lecture Series V - October 22th, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time).
Video:https://disk.pku.edu.cn:443/link/FD9BF2C2F871B7FE56EFD891777A6E7E
Valid Until:2025-01-01 00:00
Lecture 1—— Global existence and decay of solutions to Prandtl system with small analytic data
Speaker: Prof. Ping Zhang, Mathematics Institute of Academy of Mathematics and Systems Science of Chinese Academy of Sciences, Beijing
Time:2020-10-22 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
*****
Lecture Series IV - July 14th, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time)
Video: Part I:https://disk.pku.edu.cn:443/link/ADEA1AC5CB6C898F8809E6FFBC93B54C
Part II:https://disk.pku.edu.cn:443/link/8395E85B327FAC1E80993F79F2CACCF2
Valid Until:2025-01-01 00:00
Lecture 1 - WORD MAPS ON SIMPLE ALGEBRAIC GROUPS AND RELATED TOPICS
Speaker: Prof. Nikolai Gordeev, Saint Petersburg State Pedagogical University
Time:2020-07-14 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract:(/upload/editor/file/20200711/11112603683.pdf)

Lecture 2 - Structure of Commutator Subgroups
Speaker: Prof. Zuhong Zhang, Beijing Institute of Technology
Time:2020-07-14 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract:
This talk is based on the joint works with R. Hazrat, A. Stepanov and N. Vavilov in the past decade. In his seminal paper, more than half a century ago, Hyman Bass initialed the study of commutator subgroups and commutator formulas over rings. Since then, it attracted great attend of many leading experts including A. Bak, A.A. Suslin, L.N. Vaserstein, etc.. Various commutator formulas have been obtained in stable and non-stable settings and for a range of classical and algebraic like-groups.
In this talk, we will describe some recent results on the study (higher/birelative) commutators in general linear groups $GL(n,A)$ as well as their elementary generators. we will also discuss some further related research and applications.
Lecture Series III - June 30th, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time)
Download Slides:
On the way to do so, we will address the parallels between topological dynamics and ergodic theory, and their applications to combinatoric number theory.
Bio: Prof. Ye got his Ph. D. in Mechanics and Mathematics Department of Moscow State University in 1991, and then did postdoc work in ICTP during 1991-1993. He was a faculty of Mathematics School in USTC since 1993. He was selected as a member of the Chinese Academy Sinica in 2019. His research interests include topological dynamics, ergodic theory and combinatoric number theory.
******
Lecture Series II - June 16th, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time)
Video:Part I:https://disk.pku.edu.cn:443/link/F3034E87D1FC25E824BBDB14302AB24E
Part II:https://disk.pku.edu.cn:443/link/E55DFDEE3CA697F5C15F978B04ABC727
Valid Until:2025-01-01 00:00
Lecture 1 - Multiple structures for quasilinear equations by the variational method
Speaker: Alexander Nazarov, PDMI RAS and Math&Mech Faculty, St. Petersburg State University
Time:2020-06-16 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract:
We study entire bounded solutions to the equations of variational nature. The model example here is $\Delta u - u + u^3 = 0$ in $\mathbb R^2$. Our approach is purely variational and is based on concentration arguments and symmetry considerations. This method allows us to construct in an unified way several types of solutions with various symmetries (radial, breather type, rectangular, triangular, hexagonal, etc.),both positive and sign-changing. It is also applicable for more general equations in any dimension.
The talk is based on the joint paper Lerman L.M., Naryshkin P.E., Nazarov A.I., Abundance of entire solutions to nonlinear elliptic equations by the variational method, Nonlinear Analysis -- TMA. 190 (2020), DOI 10.1016/j.na.2019.111590, 1-21.
Lecture 2 - Periodic and quasi-periodic solutions of 1-d Q-curvature equation.
Speaker: Jiang Meiyue, School of Mathematical Sciences, Peking University
Time:2020-06-16 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract(/upload/editor/file/20200609/09145940157.pdf):
******
Lecture Series I - June 2nd, 2020 (20:00-22:00 Beijing time or 15:00-17:00 St Petersburg time)
Lecture 1 - Spectral synthesis for systems of exponentials and reproducing kernels
VIDEO: https://disk.pku.edu.cn:443/link/62236134E8043AD5871A88D2BA3F2868
Expiration Time:2025-07-31 23:59
Speaker:Anton Baranov (Saint Petersburg State University)
Time:2020-06-02 20:00-21:00 Beijing time (15:00-16:00 St Petersburg time)
Abstract: Let $x_n$ be a complete and minimal system of vectors in a Hilbert space $H$. We say that this system is hereditarily complete or admits spectral synthesis if any vector in $H$ can be approximated in the norm by linear combinations of partial sums of the Fourier series with respect to $x_n$. It was a long-standing problem whether any complete and minimal system of exponentials in $L^2(-a,a)$ admits spectral synthesis. Several years ago Yu. Belov, A. Borichev and myself gave a negative answer to this question which implies, in particular, that there exist non-harmonic Fourier series which do not admit a linear summation method. At the same time we showed that any exponential system admits the synthesis up to a one-dimensional defect. In the talk we will also discuss related problems for systems of reproducing kernels in Hilbert spaces of entire functions (such as Paley-Wiener or Fock).
Lecture 2 - On gauged linear sigma model and related problems
VIDEO:https://disk.pku.edu.cn:443/link/5034D664212B1B1DD783D05CA07B343D
Expiration Time:2025-07-31 23:59
Speaker: Prof. Huijun Fan (Peking University)
Time:2020-06-02 21:00-22:00 Beijing time (16:00-17:00 St Petersburg time)
Abstract: Gauged linear sigma model was proposed by Witten in the early of 90's to explain the mirror symmetry phenomenon and the CY/LG correspondence conjecture. In this lecture, I will firstly formulate the mathematical framework of the GLSM, and then describe an algebraic way to construct the quantum invariants of GLSM in narrow case (for general gauge group) via quasimaps.
This was a joint work with Jarvis and Ruan. Finally I will report the recent progress in this field and related problems.